Graphene is one of the several forms of carbon known as its “allotropes”. Allotropes are structurally different forms of the same element, in which the same atoms bond together in different ways. In the case of carbon, aside from soot and charcoal, the most commonly known forms are diamond, graphite, and the fullerene. In diamonds, the atoms are arranged in a pyramid shaped lattice. The atoms of graphite are sheets of hexagonal lattice, while fullerenes are similar lattices arranged into shapes such as balls (Buckminster fullerene) or cylinders (carbon nanotubes). The different forms have different properties: diamond is electrically insulating and hard; graphite is an electrical conductor and is soft – hence it is used as pencil “lead”.

Like graphite, graphene's atoms are arranged in a hexagonal lattice. What distinguishes it is, that rather than being made of stacked layers, graphene is one single layer just one atom thick.

There are several ways graphene can be produced. The method used by Andre Geim was to peel layers away from graphite using a sticky tape until he had a single layer [1]. It's also possible to “grow” graphene on other substances such as silicon carbide, or on metals [2]. Some of the most important properties of the material have been found in this way.

Graphene is one of the strongest materials known. It conducts heat better than diamond, and may conduct electricity better than silver. As it is two-dimensional, it could be used to detect single molecules of a gas – if a gas molecule were to stick to a sheet of graphene, there would be a local change in the electrical resistance. This could also be useful for detecting microbes.

Many of the proposed applications of graphene are in electronics and computing. Its electronic properties mean that, it could be used to make transistors for high-speed electrical circuits, and ultimately replaces silicon in microchips.

At the same time, research in China discovered that, graphene has some antibacterial properties and is effective in killing E. Coli bacteria, leading to suggestions for its use in hygiene products [3].

In the atomic ground state, the 6 electrons of Graphene are in the configuration 1s² 2s² 2p² , i.e. 2 electrons fill the inner shell 1s and 4 electrons occupy the outer shell of 2s and 2p orbitals. Because the 2p orbitals (2p_x , 2p_y , and 2p_z )  are roughly 4eV higher than the 2s orbital, it is energetically favourable to put 2 electrons in the 2s orbital and only 2 of them in the 2p orbitals (Figure 1). However in the presence of other atoms, such as H, O, or other C atoms, it is favourable to excite one electron from the 2s to the third 2p orbital, in order to form covalent bonds with the other atoms. The gain in energy from the covalent bond is indeed larger than the 4eV invested in the electronic excitation. The excited state, therefore has four equivalent quantum-mechanical states, |2s>, |2p_x >, |2p_y >, and |2p_z >. A quantum-mechanical superposition of the state  |2s> with n |2p> states is called spⁿ hybridization, which j play an essential role in covalent carbon bonds [4] .

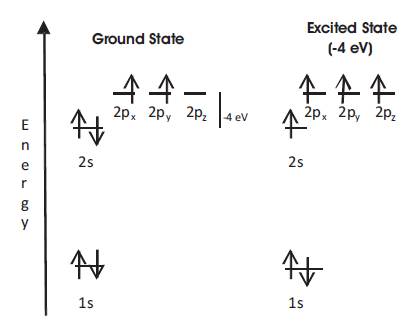
Figure 1. Electronic Configurations for Carbon in the Ground State (left) and in the Excited State (right) [4].

In the case of superposition of the 2s and two 2p orbitals, which may be the |2p_x > and the |2p_y > states, one obtains the planar sp² hybridization [5]. These orbitals are oriented in  the xy-plane and have mutual 120^o angles (Figure 2 (a)). The remaining unhybridized 2p_z orbital is perpendicular to  the plane as shown in Figure 2 (b).

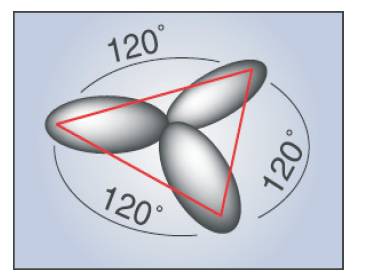
Figure 2 (a). σ orbital (in plane) derived from s, p_x , p_y

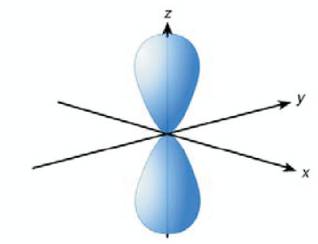
Figure 2 (b). π orbital ( to plane) derived from p

The π-bonds hybridize together to form the π-band and π∗- bands. These bands are responsible for most of graphene's notable electronic properties, via the half-filled band that permits free-moving electrons [6].

The unique electrical properties of graphene come from its electronic configuration, which is rather different from usual three-dimensional materials. Its Fermi surface is characterized by six double cones (Figure 3). In intrinsic m(undoped) graphene, the Fermi level is situated at the connection points of these cones. Since the density of states of the material is zero at the Fermi level, the electrical conductivity of intrinsic graphene is quite low. The Fermi level can however be changed by an electric field so that, the material becomes either n-doped (with electrons) or pdoped (with holes), depending on the polarity of the applied field [7].

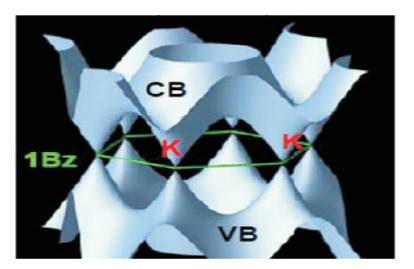
Figure 3. Fermi Surface of Graphene characterized by Six Double Cones

For electrons, energy is given by the expression,

(1)

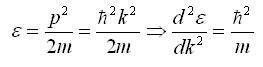
Here,

ε - electron energy,

p - momentum,

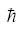=1.05X10^-34 is the reduced Plank's constant, and

k - wave number, which is defined by the following expression,

(2)

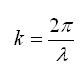
where π - constant,

λ - wave length

Thus, the electron behaves like a particle with an effective mass of,

(3)

Now at the Fermi points,

(4)

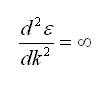
As a consequence, at the Fermi level, the electron behaves as zero effective mass particles (massless Dirac fermions). Hence, Graphene's electrical conductivity is unparalleled at room temperature, higher than silver (the least resistive metallic material).

In condensed matter physics, the Schrodinger equation rules the world, usually being quite sufficient to describe the electronic properties of materials. But, Graphene is an exception. Due to zero effective mass of the electrons, they  attain a speed of approximately c_g ~10⁶ m/s. Thus the g charge carriers mimic relativistic particles, and are more easily and naturally described starting with the Dirac equation rather than the Schrodinger equation [8] .

Graphene is the strongest material ever tested, [9] with an intrinsic Tensile strength of 130 GPa and a Young's modulus (stiffness) of 1 TPa (150000000 psi) [10]. It is said that, 1 square meter graphene hammock would support a 4 kg cat, but would weigh only as much as one of the cat's whiskers, at 0.77 mg (about 0.001% of the weight of 1 m² of paper) [10]. Graphene's stability is due to its tightly packed carbon atoms and the sp² orbital hybridization. Resulting σ bonds provide exceptional structural rigidity. The carbon–carbon bond length in graphene is about 0.142 nanometers [9].

Devices such as plasma TVs and phones are commonly coated with a material called indium tin oxide. Manufacturers are actively seeking alternatives that could cut costs and provide better conductivity, flexibility, and transparency. Graphene is non-reflective and appears very transparent. Its conductivity also qualifies it as a coating to create touch screen devices. As graphene is both strong and thin, it can bend without breaking, making it a good match for the bendable electronics devices.

Another fascinating property of Graphene is related with its softness. Because the material is only one atom thick, it can be easily deformed in the direction normal to its surface. The sigma bonds in Graphene are extremely exclusive and directional and hence do not easily accept the foreign atoms. Hence, natural graphene (that is, graphene in the form of graphite) is a very pure material with no extrinsic disorder.

Gas molecules that land on Graphene affect its electronic properties in a measurable way. This means that gas sensors could be created which are sensitive to a single atom or molecule.

Graphene is one of the strongest materials known. It conduct electricity better than silver. As it is twodimensional, it could be used to detect single molecules of a gas – if a gas molecule were to stick to a sheet of graphene, there would be a local change in the electrical resistance. This could also be useful for detecting the microbes.

Many of the proposed applications of graphene are in electronics, touch screens and computing. Its electronic properties mean it could be used to make transistors for high-speed electrical circuits, and ultimately replace silicon in microchips.

Acknowledgement

The work is accomplished with the help of resources of Department of Physics, DAV College, Amritsar, India.