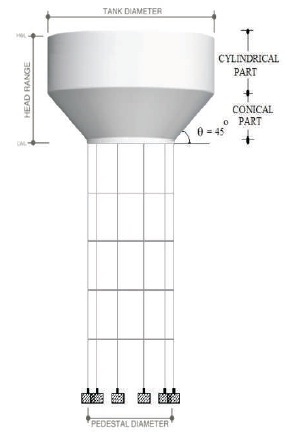
Figure 1. Reinforced Concrete Combined Conical Elevated Water Tank for Present Study
Combined conical elevated water tanks are widely used as water reservoirs in various locations around the world. Current Indian codes of practice do not provide any provisions or guidelines for the analysis and design of reinforced concrete combined conical elevated water tanks under hydrostatic loading. Available codes provisions are limited only for the design of cylindrical and rectangular tanks. The present study investigates the behavior of combined conical elevated water tank under varying water percentage in conical and cylindrical parts by performing dynamic analysis i.e. Response Spectrum method. The main aim of this research work is to find out the optimum water percentage in conical and cylindrical part of combined conical elevated water tank. The modeling and analysis of combined conical elevated water tank are carried out by using structural analysis and design computer program, i.e. STAAD.Pro. Total twenty numbers of models were made for empty tank and full tank condition, where these models are provided with ten numbers of columns along with the periphery of a circle with five staging level and connected by using Cross Staging pattern. The response of each combined conical elevated water tanks are represented graphically.
Elevated water tanks are extensively used for storing various liquids in municipalities and industries. The commonly used shapes for these tanks are rectangular and circular. Now-a-days truncated conical shape type tanks are commonly used for storing liquids in various locations around the world. The truncated conical water tank is divided into two types “combine conical tank” and “pure conical tank”. A conical vessel with a top overlying cylindrical part is referred as a “combine conical tank” whereas a conical vessel without top overlying cylindrical part is referred as a “pure conical tank” as shown in Figure 1. The top conical part is made of either Steel or Reinforced concrete while the shaft is usually made of by Reinforced concrete. The current study focuses on the seismic behaviour of combined conical elevated water tank under water percentage in conical part of a constant volume of 500 m3.

Figure 1. Reinforced Concrete Combined Conical Elevated Water Tank for Present Study
The present study investigates the behavior of combined conical elevated water tanks, following are some literature that are considered in the present study. Damatty et al. (1997) carried out a study to evaluate the stability of imperfect steel conical tanks under hydrostatic loading. Conical steel tank was modeled by using Finite element shell that includes both geometric nonlinearities and material nonlinearities. The result of their study showed that liquid filled conical shells are quite sensitive to geometric imperfections. This simplified approach was again extended by Damatty et al. (1998) to investigate the effect of different imperfection shapes on the inelastic stability of liquid filled conical tank. The result of their study showed that inelastic buckling of steel conical tanks was most sensitive to axisymmetric imperfection. Damatty et al. (2001) found that the use of welded stiffeners at the bottom portion of steel conical tank can significantly improve the buckling capacity of conical tanks.
This simplified approach of the stiffener is again considered by Damatty and Marroquin (2002) for existing tanks and for the design of newly stiffened ones. The design procedure is then described graphically. Later on, Sweedan and Damatty (2002) carried out an experimental and analytical study for the dynamic characteristics of conical shells. The results of their study are very useful in assessing the dynamic response of conical tanks when it is subjected to wind load and seismic forces. Sweedan and Damatty (2005) carried out a study on Equivalent models under vertical ground excitation. The current study is conducted to establish a simple design procedure that can be used to estimate the seismic forces acting on conical tank subjected to vertical ground excitation. Damatty et al. (2005a) perform an experimental study conducted on a liquid filled combined conical tank. The results of these experiments are used to validate the assumption of previously developed analytical model for the free sloshing motion, and similar experiments are again conducted by Damatty et al. (2005b) for knowing the dynamic characteristic of conical vessel and evaluation of wind forces and seismic forces. Damatty and Sweedan (2006) have developed a simplified mechanical analog which can be used to estimate the forces associated with horizontal ground excitation. The proposed mechanical analogue considers both the components, i.e. Impulsive and convective component of hydrodynamic pressure. Sweedan and Damatty (2009) have carried out a study which covers the design approach for combined steel conical tank and to provide safety against hydrostatic loading and buckling. Hafeez et al. (2010) have carried out a study on combined imperfect conical tanks subjected to hydrostatic loading and conclusion are drawn between buckling capacities of combined tanks to equivalent pure conical tanks. Hafeez et al. (2011) carried out an analysis on stability of conical tank against the combined effect of wind loading and hydrostatic pressure on the conical tank. Elansary and Damatty, (2013) have carried out a Non-linear finite element analysis on a reinforced concrete conical tank in order to understand their behavior under hydrostatic pressure, where the model of the conical tank is made of by using shell elements. Jolie et al. (2013) carried out a study for assessment of current design procedures for conical tank by using previously established equivalent model under horizontal excitation. The results of their study reveal that the approximate approach is not adequate for designing conical tanks to resist the seismic forces. Using the same equivalent mechanical model Jolie et al. (2014) have performed the seismic analysis of pure conical tanks under vertical excitation to estimate the normal forces developed in the tank walls. In addition to this, a three-dimensional finite element model is developed to predict the maximum membrane and meridional stresses developed due to both hydrostatic and hydrodynamic pressure distributions. Azabi (2014) had analysed a set of reinforced concrete elevated pure conical tanks using a finite element method based on a three-dimensional consistent shell element developed by Koziey and Mirza, Azabi then assessed the accuracy of a simplified approach for the analysis and design of reinforced concrete conical tanks. The comparison in carried out between the internal force obtained from their numerical models with those resulting from the Portland Cement Association (PCA) design aids, a significant difference was obtained between the internal force from their numerical models with those resulting from the Portland Cement Association (PCA) design aids. The Finite element model made by Azabi (2014) was extended by EIansary et al. (2015) to account the shrinkage and nonlinear behavior of concrete under hydrostatic pressure. Total twelve reinforced concrete finite element model were made with a wide range of practical dimensions. The results of their study were concluded for maximum deflection of tank’s wall, Maximum hoop stress and Maximum meridional stress are considered. Elansary et al. (2016) developed a nonlinear Finite Element Model (FEM) based on shell element, used to analyze reinforced concrete conical tanks subjected to hydrostatic pressures. They also replaced the conical vessels with an equivalent cylinder. The result of the equivalent cylinder is compared with the finite element model, where the model is used to develop the charts for adequate thickness and straining action that developed in to a liquid filled reinforced concrete conical tank. Musa and Damatty (2016) have performed a static pushover analysis on a finite element model of steel conical tank subjected to hydrodynamic pressure associated with vertical ground excitations. They have also considered the effect of geometric and material nonlinearities and finally the charts are developed to estimate the capacity of steel conical tanks to resist the vertical ground excitation. Elansary and Damatty (2017) have presented the first comprehensive study conducted on liquid filled composite conical tank. A finite element model of composite conical tanks was made considering both geometric and material nonlinearity. The variations of hoop stresses and meridional stresses along the thickness of the tanks are determined and the stresses at the outer faces of the tank’s wall are plotted. They concluded that maximum hoop stresses in concrete occur in 0.15 to 0.3 heights of the tank and maximum meridional stresses occur at 0.1 heights of the tank.
A reinforced concrete combined conical elevated water tanks are considered for the present study with a constant volume of 500 m3. A set of total twenty tanks were modeled by varying water percentage in conical portion using structural analysis and design computer program, i.e. STAAD.Pro. The water in conical part is increased at a rate of 5% of the overall volume, in conical part, and all the tanks were model for Seismic Zone-V as per IS-1893:2002. These tanks are provided with ten numbers of columns with cross staging patterns. The conical and cylindrical portion of the tanks were modeled by using four nodes iso-parametric plate element whereas the columns and ring beams/staging beams are modeled as beam element with six degree of freedom at each node. All the tanks are provided with fixed support conditions shown in Figure 2 and 3.
Figure 2. Combined Conical Elevated Water Tank under varying Water Percentage in Conical Portion and Cylindrical Portion (Percentage of Water in Conical Part varies from 5% to 50%)
Figure 3. Combined Conical Elevated Water Tank under varying Water Percentage in Conical Portion and Cylindrical Portion (Percentage of Water in Conical Part varies from 55% to 100%)
Modeling of combined conical elevated water tanks using computer program, i.e. STAAD.Pro is done by assuming preliminary geometrical properties shown in Table 1, are used for the analysis of combined conical elevated water tanks. The sizes of each tanks were chosen for a constant volume capacity of 500 cubic meter.
As per Indian Standard codes such as IS-1893:2002 Part-II provides the guideline for Earthquake resistant design of liquid retaining structure, IS-3370:2009 (Part-I to IV) provides the guideline for design of concrete structure storing liquid, and IS-11682:1985 provides the guideline for design of RCC staging for overhead water tanks. Tables 2 and 3 show the design parameter and sectional properties, which were used in the analysis of combined conical elevated water tanks.

Table 2. Design Parameters for Combined Conical Elevated Water Tank
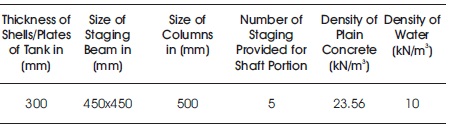
Table 3. Sectional Properties of Combined Conical Elevated Water Tank
The static and dynamic analysis of combining conical elevated water tanks is carried out by using computer program, i.e. STAAD.Pro. The analysis of combined conical elevated water tanks is separately carried out for empty and full tank conditions and the result obtained from computer program for empty and full tank condition is shown in Tables 4 and 5.
Table 4. Maximum Values of Base Shear, Axial Force, Moments, and Lateral Displacement for Empty Tank Condition
Table 5. Maximum Values of Base Shear, Axial Force, Moments, and Lateral Displacement for Full Tank Condition

Graph 1. Values of Base Shear for Empty Tank Condition
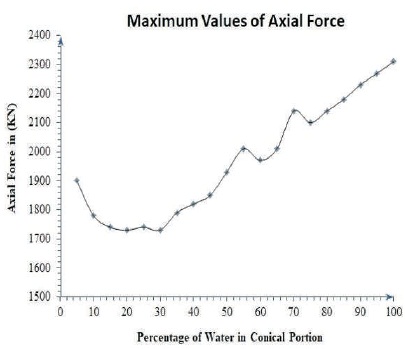
Graph 2. Value of Axial Force for Empty Tank Condition
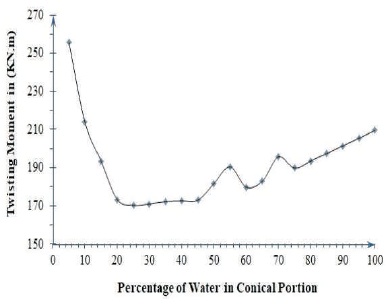
Graph 3. Values of Moment in x-direction for Empty Tank Condition
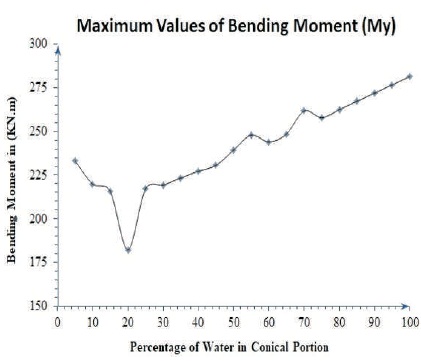
Graph 4. Values of Moment in y-direction for Empty Tank Condition

Graph 5. Values of Moment in z-direction for Empty Tank Condition
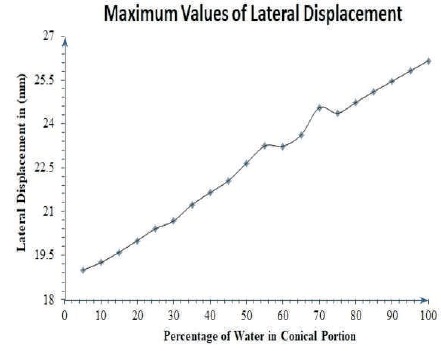
Graph 6. Values of Lateral Displacement for Empty Tank Condition
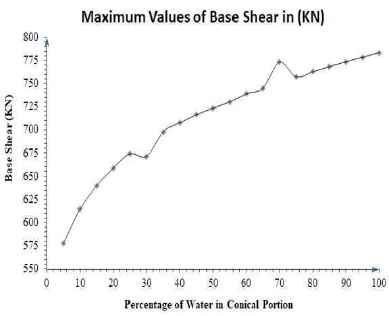
Graph 7. Values of Base Shear for Full Tank Condition
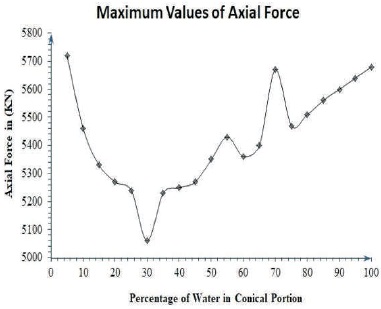
Graph 8. Value of Axial Force for Full Tank Condition
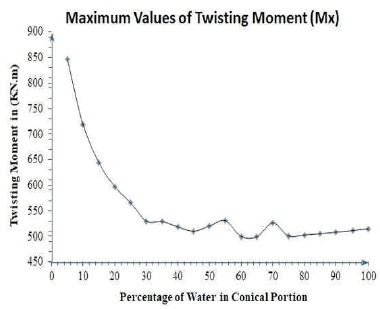
Graph 9. Values of Moment in x-direction for Full Tank Condition
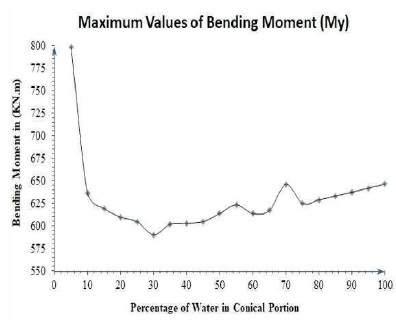
Graph 10. Values of Moment in y-direction for Full Tank Condition
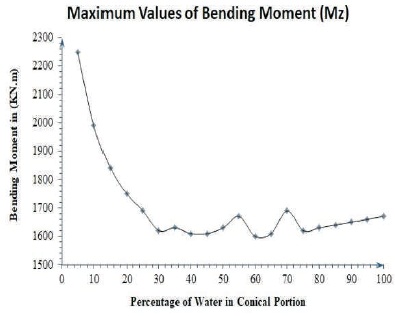
Graph 11. Values of Moment in z-direction for Full Tank Condition
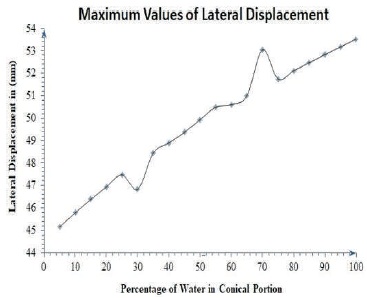
Graph 12. Values of Lateral Displacement for Full Tank Condition
From the above discussion, it is concluded that