Many statistical measures used in education are experimental research designs that require strict scientific methodologies. Many of these methods cannot be implemented in educational institutions without violating legal policies or severely disturbing the learning environment. The time has come for education to provide its own scientific field that has its own measures. This novel field should be grounded in longstanding educational research, fundamental educational theory, and innovations in qualitative, quantitative, and mixed methods research designs native to the specifics of pedagogy and andragogy. This paper provides a definition for the establishment of this new field called: “Education Science”. A comprehensive statistical test for the field of Education Science is Trichotomy–Squared.

Education Science

The field of “Education Science” is also represented by the term “Eduscience” which is a portmanteau of the two terms “Education” and “Science”. Similar to the field of “Bioscience”, Eduscience is the study of education wherein applicable sciences (such as ergonomics, statistics, technology, etc.) are applied to enhance and improve learning. The primary purpose of the field of Eduscience is the study and application of solutions to improve and enhance the learning environment and learning in general. Eduscience is solution–driven and is actively concerned with the transfer and dissemination of knowledge.

Education Science is a broad field and its professionals are directly involved in the field. Those who are actively involved in Eduscience can be referred to as “Education or Educational Scientists”. Educational Scientists or “Eduscientists” are multifaceted professionals in a variety of areas of expertise. They can assume many or multiple roles in the educational environment and can serve in a variety of offices or in a multitude of capacities. The primary positions in the learning environment that Eduscientists assume are in the following areas: Administration (which includes Leaders, Organizational Heads, and Organizational Management Professionals), Instruction (which includes Teachers, Professors, and Facilitators), Practice (which includes Practitioners in a variety Specified Areas and Arenas), and Technology (which includes Educational Technologists, Instructional Technologists, and Information Technologists). In these positions Eduscientists effectively use, analyze, study, and deploy novel instructional and learning theories, methodologies, strategies, solutions, tools, and techniques in both traditional or virtual pedagogical and adragogical settings to bring about learning. Educational Scientists strive to make the process of knowledge transfer transitive. A transitive knowledge transfer process is seamless and as harmonious as possible in an effort to empower, enhance, and improve learning.

Eduscientists are masters of teaching who also are also highly proficient practitioners who are able draw from personal and professional experiences to make the learning environment more viable (Accessible), usable (Ergonomic), teachable (Instructional), engaging (Relevant), approachable (Adaptive), exploration–based (Discovery), and inspirational (Transformative). The Total Transformative Trichotomy–Squared Test is a comprehensive multi–step research methodology that is employed by Eduscientists. It is especially designed to conduct qualitative and quantitative investigations in education.

“Trichotomy” Origins

The term is pronounced ['trahy-kot-uh-mee'], spelled “trichotomy”, and is a noun with the plural written form “trichotomies”. A “Trichotomy” in terms of philosophy can be referred to as a threefold method of classification. Philosopher Immanuel Kant adapted the Thomistic acts of intellect in his trichotomy of higher cognition — (a) understanding, (b) judgment, (c) reason — which he correlated with his adaptation in the soul's capacities — (a) cognitive faculties, (b) feeling of pleasure or displeasure, and (c) faculty of desire — of Tetens's trichotomy of feeling, understanding, will. (Teo, 2005). In terms of mathematics, Apostol in his book on calculus defined “The Law of Tricohotomy” as: Every real number is negative, 0, or positive. The law is sometimes stated as “For arbitrary real numbers a and b, exactly one of the relations a < b, a = b, and a > b holds” (Apostol, 1967)

It is important to note that in mathematics, the law (or axiom) of trichotomy is most commonly the statement that for any (real) numbers x and y, exactly one of the following relations holds. Until the end of the 19th century the law of trichotomy was tacitly assumed true without having been thoroughly examined (Singh, 2002). A proof was sought by Logicians and the law was indeed proved to be true. If applied to cardinal numbers, the law of trichotomy is equivalent to the axiom of choice. More generally, a binary relation R on X is trichotomous if for all x and y in X exactly one of xRy, yRx or x = y holds. If such a relation is also transitive it is a strict total order; this is a special case of a strict weak order. For example, in the case of three elements the relation R given by aRb, aRc, bRc is a strict total order, while the relation R given by the cyclic aRb, bRc, cRa is a non–transitive trichotomous relation. In the definition of an ordered integral domain or ordered field, the law of trichotomy is usually taken as more foundational than the law of total order, with y = 0, where 0 is the zero of the integral domain or field. In set theory, trichotomy is most commonly defined as a property that a binary relation < has when all its members satisfy exactly one of the relations listed above. Strict inequality is an example of a trichotomous relation in this sense. Trichotomous relations in this sense are irreflexive and antisymmetric (Sensagent, 2012).It is from these logical and mathematical definitions that the author derives the definition of “Research Trichotomy” and applies it to the qualitative and quantitative analysis of the affective domain of learning.

The term “Trichotomy” is defined in Trichotomy–Squared in the following manner: “Trichotomy”: is pronounced ['trahy-kot-uhmee'], spelled “trichotomy”, and is a noun with the plural written form “trichotomies”. “Trichotomy” has the following threefold definition: (1) Separation or division into three distinct parts, kinds, groups, units, etc.; (2) Subdivision or classification of some whole into equal sections of three or “trifold segmentation”; and (3) Categorization or division into three mutually exclusive, opposed, or contradictory groups, for example – “A trichotomy between thought, emotions, and action.”

Tri–Squared Foundations

Tri–Squared comprehensively stands for the “Total Transformative Trichotomous–Squared Test” (or “Tri–Squared”). The Total Transformative Trichotomous–Squared Test provides a methodology for the transformation of the outcomes from qualitative research into measurable quantitative values that are used to test the validity of hypotheses. The advantage of this research procedure is that it is a comprehensive holistic testing methodology that is designed to be static way of measuring categorical variables directly applicable to educational and social behavioral environments where the established methods of pure experimental designs are easily violated. The unchanging base of the Tri–Squared Test is the 3 × 3 Table based on Trichotomous Categorical Variables and Trichotomous Outcome Variables. The emphasis on three distinctive variables provides a thorough and rigorous robustness to the test that yields enough outcomes to determine if differences truly exist in the environment in which the research investigation takes place.

Tri–Squared is grounded in the combination of the application of the research by two mathematical pioneers and the author's research on two dimensional foundational and three dimensional Instructional Design. The aforementioned pioneers in mathematical research includes the original dissertation of optical pioneer Ernst Karl Abbe who derived the distribution (Abbe, 1863) that would later become known as the chi square distribution and the original research of mathematician Auguste Bravais who pioneered the initial mathematical formula for correlation in his research on observational errors (Bravais, 1846).. The Tri–Squared research procedure uses an innovative series of mathematical formulae that do the following as a comprehensive whole: (1) Convert qualitative data into quantitative data; (2) Analyze inputted trichotomous qualitative outcomes; (3) Transform inputted trichotomous qualitative outcomes into outputted quantitative outcomes; and (4) Establish an effect size and corresponding sample size with an associated alpha level to test the validity of an established research hypothesis.

The basis of Tri–Squared is similar to the Meta–Cognitive Analysis method of Snell and Marsh that was designed to replace Meta–Analysis as a more effective means analyzing data in the social and behavioral sciences (Snell & Marsh, 2003). Interestingly enough, the Chi–Square Test that is used in Meta–Cognitive Analysis was implemented by Karl Pearson who credited Ernst Karl Abbe with the original discovery of the mathematics of that led to the Chi Square distribution and the subsequent test. Tri–Squared differs from Chi–Square in its complete use of trichotomy as criterion values. It also differs in its infusion of the sample size and subsequent alpha level in its initial calculations (thereby enabling the educational scientist to calculate effectively and determine statistical significance rapidly based of off the initial research conditions).

To effectively use Tri–Squared in a research investigation one must first develop a series of “trichotomous categorical variables” based on associated “trichotomous outcome variables”. This is the first initial and crucial step to using Tri–Squared as a valid, reliable, and objective means of analyzing data. Second, a specific “trichotomy-engineered” or “Inventive” (i.e., original) Investigative Instrument must be created and implemented based on the initial trichotomous categorical variables and outcomes. This insures that the research investigation is consistent throughout the study and that the later Tri–Squared computations are validly reporting what actually took place in the research environment. The “Inventive Investigative Instrument” can be psychometrically delivered as a test, qualitatively delivered in the form of a research questionnaire, provided anonymously as a survey, given as in–depth questions during an interview, or evaluated as a comprehensive metric via an assessment rubric. As long as the trichotomous categorical variables are measured according to the established associated trichotomous outcome variables then the research has merit within the strict confines and rigorous requirements of the Tri–Squared Test.

The Tri–Squared statistic transforms qualitative data into quantitative data and then measures the difference between the two to determine the validity of the initial research hypothesis. If no relationship is determined to exist between the qualitative and quantitative trichotomous categorical and outcome variables, then the Tri–Squared statistic equals zero. Adversely, the greater the relationship between the variables, the greater the value will be of the final Tri–Squared calculation. Statistics by nature is a methodical and selective science that requires specific and precise steps and it is cautious when dealing with uncertainty. Thus, Tri–Squared is very comprehensive in its structure taking into account: an exact number of measures, rigorous mathematical accountability through trichotomous metrics, precision in determining the research study effect size, specification in determining the ideal research sample size, and strict methodology in hypothesis testing.

The Tri–Squared distribution is a static mathematical extraction out of the Chi Square distribution. This test is not the only test based on the Chi Square distribution (as it is a mathematical distribution that is frequently used directly or indirectly in many tests of significance). Similar to the Chi Square distribution the Tri–Squared distribution has the following characteristics: (1) It has only a single parameter (the distribution Degrees of Freedom written as “d.f.”); (2) The entire distribution is positively skewed; and (3) The Degrees of Freedom are mathematically written, “[C – 1][R – 1]” which is equal to the distribution mean. Unlike, the Chi Square distribution the Tri–Squared distribution has the following characteristics: (1) The distribution Degrees of Freedom never changes, therefore, it never approaches the Normal Gaussian Distribution (the bell curve); (2) As a static test the Tri–Squared Degrees of Freedom is always [C – 1][R – 1] = [3 – 1][3 – 1] = [2][2] = 4 = the distribution mean; (3) The distribution mode is always [d.f. – 2] = [4 – 2] = 2; (3) The distribution median is always approximates [d.f. – 0.7] = [4 – 0.7] = 3.3; (4) Due to the static or unchanging nature of the distribution, the distribution skew is always positive with the d.f. always equaling 4; and (5) The distribution formulae uses brackets “[ ]” (similar to the science of chemistry) in its formulaic notations to emphasize and imply “concentration” or “a concentration on” for purposes of clarity. The Tri–Squared distribution is the foundation for the Tri–Squared Test which comprehensively incorporates the following Tri–Squared formulae: The Calculated Column Standard Deviation, The Calculated Row Standard Deviation, and The Sample Effect Size. The Tri–Squared Test is designed to create a comprehensive holistic research methodology from calculations conducted on the Standard 3 × 3 Tri–Squared Table which produces the following: (1) A positive result; (2) No information on the variable relationship direction; and (3) Associated Effect Size, Sample Size, and Alpha Levels.

Step One: Design of an Inventive Investigative Instrument that has Trichotomous Categorical Variables and Trichotomous Outcome Variables.

To effectively use Tri–Squared in a research investigation the researcher must first develop a series of trichotomous categorical variables based on associated trichotomous outcome variables. This is the first initial and crucial step to using Tri–Squared as a valid, reliable, and objective means of analyzing data. Second, an “Inventive Investigative Instrument” must be created and implemented in a manner compliant with the initial trichotomous categorical variables and outcomes stated at the outset of the research investigation. This insures that the research investigation is consistent throughout the study and that the later Tri–Squared computations are validly reporting what actually took place in the research environment during the time period in which the actual study was conducted. As previously stated the “Inventive Investigative Instrument” can be psychometrically delivered as a test or qualitatively delivered in the form of a research questionnaire, survey, interview or another type of data acquiring metric. As long as the trichotomous categorical variables are measured according to the established associated trichotomous outcome variables then the research has merit within the confines and strict requirements of the Tri–Squared Test.

Step Two: Establish the Research Effect Size, Sample Size with associated Alpha Level.

The Tri–Squared Effect Size Comprehensive Analytical Mathematical Formula

(1)

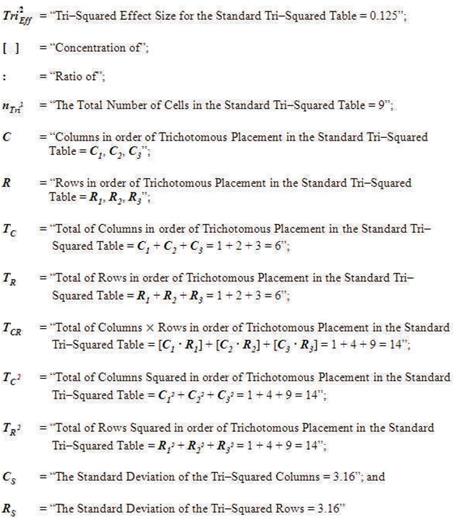
The Comprehensive Mathematical Formula of the Standard Deviation for the Standard 3 × 3 Tri–Squared Table Columns

(2)

The Comprehensive Mathematical Formula of the Standard Deviation for the Standard 3 × 3 Tri–Squared Table Rows

(3)

The Comprehensive Tri–Squared Formulae Definitions for the Standard 3 × 3 Tri–Squared Table

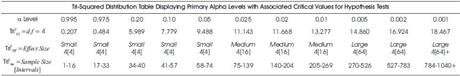
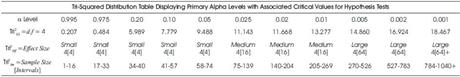
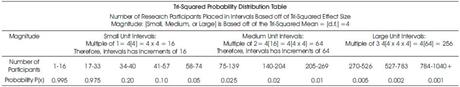
The result of the Tri–Squared Effect Size for the Standard 3 × 3 Tri–Squared Table is calculated to be 0.125. This means that an alpha level of 0.10 is the standard for Tri–Squared hypothesis test for a research investigation designed to have a small sample size (specifically identified as 41 to 57 participants for = 0.10). This is further illustrated in the following Tri–Squared Distribution Two–Tailed Mathematical Hypotheses and Standard Tri–Squared 3 × 3 Tabular Format Data Analysis Computation Methodology (Table V):

Step Three: Establish Mathematical Hypotheses.

Two–Tailed Mathematical Hypotheses for the Tri–Squared Test

(4)

Step Four: Use the Tri–Squared Test as the Data Analysis Procedure following implementation.

This is the final step in the Tri–Squared Test. An example of the research design reporting methodology follows in the Standard Tri–Squared 3 × 3 Tabular Format.

Sample Outcomes of the Computational Tri–Squared Test

Figure 1 shows the Sample Trichotomy–Squared Test illustrating the standard 3 × 3 Tri–Squared Formula and qualitative table of outcomes reporting results using the standard Tri–Squared 3 × 3 Format. Sample data analyzed using the Trichotomous–Squared Three by Three Table was designed to analyze the research questions from an Inventive Investigative Instrument with the following Trichotomous Categorical Variables: a₁ = Cognitive [Knowledge]; a₂ = Affective [Dispositions]; and a₃ = Psychomotor [Skills]. The 3 × 3 Table has the following Trichotomous Outcome Variables: b₁ = Affirmative [Yes]; b₂ = Negative [No]; and b₃ = Inapplicable [Irrelevant]. The Inputted Qualitative Outcomes are reported as follows:

Figure 1. Sample Outcomes of the Computational Tri–Squared Test

The Tri–Square Test Formula for the Transformation of Trichotomous Qualitative Outcomes into Trichotomous Quantitative Outcomes to Determine the Validity of the Research Hypothesis:

(5)

(6)

Tri² Critical Value Table = 7.779 (with d.f. = 4 at α = 0.10). The calculated Tri–Square value is 8.180, thus we can reject the null hypothesis by virtue of the hypothesis test which yields the following: Critical Value of 7.779 < 8.180 the Calculated Value.

Table One illustrates the mathematical application and statistical analysis of the Trichotomous–Squared (also referred to as “Total Transformative Trichotomy–Squared”, or more simply as “Trichotomy–Squared”, “Tri–Squared” or “Tri–Square”) procedure using sample data. “Tri–Squared” is the mathematical transformation of qualitative data into quantitative data for the purpose of validating a research hypothesis. The paragraph that immediately follows the Table reports the Table's findings (which would appear in this sample paragraph). It is important to note that a researcher is not limited to the standard calculated sample size in from the base calculation in the range of 41–57 participants and may select a larger sample size and associated alpha level based upon research design and needs. The mathematical formula for Trichotomy–Squared can be broken down into specific components or “elements” that illustrate the value of the entire trichotomous research design methodology. These same elements illustrate and validate the process of transforming qualitative data into quantitative data as a means of true in–depth mixed methods for the purposes of discrete data analysis. To provide a clearer understanding of the Trichotomous Tri–Squared Test as a Total Transformative Trichotomy the following elements of the Tri–Squared formula are provided with their specific meanings:

The Comprehensive Tri–Squared Test Mathematical Computational Formulae Definitions

Definition of the Trichotomous–Squared formula provides the foundation for a more detailed definition of the mathematical formula which is: “Trichotomy–Squared is equal to the total summation of the concentrated ratio of trichotomous qualitative outcomes with transformed transitive trichotomous quantitative outcomes subtracted from trichotomous quantitative outcomes squared.” This can be simplified into, “Tri–Squared is the total concentrated sum of the ratio of the square of the difference between inputted qualitative outcomes and associated outputted quantitative outcomes” (Figure 2).

Figure 2. A Graphical Model Representing the Standard Tri–Squared Distribution

Table 1. The Effect Size Table for the Standard 3 × 3 Table from the Tri–Squared Effect Size Formula

Table 2. The Outcome of the Tri–Squared Effect Size Formula on the Tri–Squared Effect Size Table

Table 3. The Result of the Tri–Squared Formula on the Tri–Squared Probability Distribution Table

Table 4. The Result of the Tri–Squared Formula on the Tri–Squared Alpha Level Table

Table 5. The Summative Comprehensive Tri–Squared Formula Probability Distribution

Table 6. The Tri–Squared Hypothesis Test: Alpha Level, Effect Size, and Sample Size Table

The advent of Education Science heralds a new day of independence in education. Education is no longer regulated to defining its research methods by the tools and techniques of other disciplines. Often the statistical measures that are currently in use do not match the specific needs or requirements of the educational learning environment. The Tri–Squared Test exemplifies this new movement towards research independence by effectively empowering the field of Eduscience. The Total Transformative Trichotomy–Squared Research Design Methodology is a universally applicable in–depth investigative procedure that is an ideal way of examining the unique, diverse, and many times small educational and social behavioral settings that are a part of academia. This process allows researchers to validate qualitative methods by transforming data into measurable quantitative outcomes. Both qualitative and quantitative methods are seamlessly incorporated into a unified data analysis methodology that delivers a reporting procedure that specifically aligns with the initial research objectives, hypotheses, and variables. This method of statistical analysis resolves the age old issue of choice between qualitative or quantitative research designs. It does so by seamlessly combining the two opposing branches of research for maximized statistical power. This provides Educators, Practitioners, Technologists, and Social and Behavioral Scientists with a discrete comprehensively stringent data analysis tool that is purely objective, extremely reliable, and very valid. All of the latter are critical components for the acceptance of novel research in the academy and places added value on investigative research in specified academic disciplines. Tri–Squared is designed to empower 21st Century researchers who are seeking statistical tools that validate their research. It enables investigations designed for educational institutions, specialized environments, and distinctive situations. It illustrates the value of analyzing small groups, sample sizes, and individuals without sacrificing statistical power or the value of research due to the uniqueness of the environment under examination. This novel tool effectively validates the new and emerging field of Educational Science by undergirding it in a comprehensive methodology of qualitative and quantitative data analysis. This thereby insures that future research has a well–grounded foundation from which new and innovative investigative methods can draw upon. Thus allowing researchers in the future to extend their investigations into new and groundbreaking vistas that will not only improve education but learning as a whole.