Figure 1. Schematic Drawing of the Considered Power Plant Cycle
This paper concerns the thermoeconomic analyses of a cogeneration steam power plant cycle with a total capacity of 350 MW. The exergy destruction and improvement potential of each component is calculated. Moreover, exergoeconomic factors, total cost, and exergy related costs were calculated to determine the influence of each component and to find the best way of decreasing generation costs. The methodology is based on the Theory of Exegetic cost. Thermodynamic properties of the inlet and outlet points of each component in the steam plant have been specified via the Thermax program, and economic analyses by employing both Excel and Matlab software packages. Under the considered status, the analysis shows that the maximum amount of exergy destruction and potential improvement occurs in the boiler, with 75% and 81%, respectively, and the exergetic efficiency has been 66.21% for the whole power plant cycle. The exergoeconomic factors and the total costs are calculated individually and for the whole plant cycle. The unit cost of steam, work, and cooling water for the plant are found. The unit cost of steam, work, and cooling water for the plant, in which they are found to be 0.030, 0.014, and 0.45$/kWh, respectively. Referring to the comparison with the simple power cycle under the same conditions, the steam unit cost remains mostly the same for both power cycles, while the work unit is widely different.
Depletion of fossil fuel resources and global warming are two major concerns of future conventional energy systems. Energy demand is steadily increasing while the available renewable energy resources still have technology constraints in their applications. Hence, the effort for more efficient use of conventional energy systems is growing and researchers in the field of energy conversion have prompted to seek ways to design systems with minimized costs, low energy consumption, high performance, and low environmental emissions (Ahmadi et al., 2012). Due to the continuously increasing demand for natural resources by conventional energy conversion technologies, as well as the serious concern for the impact on the environment due to global warming, waste emission and disposal researches focus on the application of a new advanced method that aids in understanding how to improve the design and operation of energy systems, as well as preventing residues from contaminating the environment.
In exergoeconomic analysis, a combination of the concept of the cost, which is an economic property, and the exergy which is an energetic property, is done to achieve the best balance between thermodynamics and economics. The production process of a complex energy system can be evaluated based on its economic profitability and efficiency concerning resource consumption (Rakesh et al., 2016). Hence, the economic analysis can calculate the cost of fuel, operation, and maintenance of the total plant or individual component. On the other hand, the thermodynamic analysis provides the efficiency of each component or overall plant and evaluate their significance in terms of the overall production process. Thus the shortcoming of thermodynamics and economic analysis is overcome by exergoeconomic analysis (Rashad & El Maihy, 2009). In this paper, we have desired to conduct the energy, exergy, exergoeconomic, and sensitivity analyses for a thermal power cycle under the considered operating conditions.
Exergoeconomic emerged as a useful tool that combines exergy analysis of the system with economic constraints, where it provides information that are not available through conventional thermodynamic and economic analyses (Sahoo, 2008). This systematic approach, therefore, allows engineers to identify the location and sources of thermodynamics losses in terms of the overall production cost. This enables them to exploit these resources effectively. By allocating costs to flow streams in each process, exergoeconomic helps in the assessment of the economic effect due to the irreversibilities. Exergoeconomic not only helps calculate the cost associated with exergy destructed and the analysis of cost formation for each component separately during the plant operation, but it can also be used in optimizing the design of the new plants and assessing rational prices of the products of such plants.
Bejan et al. (1996) have explained the fundamentals of the exergy analysis and entropy generation minimization, economic and exergoeconomic analyses. Their work reviews many concepts, like the irreversibility, entropy generation, or exergy destruction, where the exergy flows and accumulates in the closed and open systems with heat transfer processes, and in power and refrigeration plants. Ahmadi et al. (2011) have performed thermodynamic modeling, exergy and exergoeconomic analyses, and optimization techniques. Their results confirm that the highest exergy loss related to the combined cycle plants occurs in the steam boiler. This is attributed to the high irreversibility process during the combustion development and due to the excessive operating temperature difference. Exergoeconomic analyses have shown that the greatest exergy loss cost is realized in the combustion chamber. They also emphasized that the rise in the input heat to the gas turbine in a recovery system creates a decreasing effect on the exergy loss cost of the whole plant.
Manesh et al. (2014) determined the optimum integration of a steam power plant, including a source and site utility system as a sink for the steam and power productions. This has been done by employing the exergy, exergoeconomic, and exergo environmental analyses. The results indicates that this type of integration represents an advantageous option from the exergetic, economic, exergoeconomic, and exergoenvironmental viewpoints.
Bolatturk et al. (2015) has performed a thermodynamic and exergoeconomic analyses of the Cayirhan Thermal Power Plant. They found out the thermodynamic properties at each and every point of the studied steam flow cycle by means of the engineering equation solver software package. Employing the obtained thermo-dynamic properties, the first and second law efficiencies were found to be 38% and 53%, respectively. The exergy destruction, improvement potential, and exergoeconomic factor were determined for each component in the plant. The maximum exergy destruction occurs in the boiler, and hence the improvement potential is the largest for the boiler. The exergoeconomic factor, has been maximum for the turbine group, followed by the boiler and finally the condenser. The low value of the exergoeconomic factor for the boiler leads to more exergy destruction to be occurring, and hence the improvement can be done by reducing the exergy destruction associated with an increase of the investment on the boiler.
Gupta and Kumar (2015) have performed the thermoeconomic optimization of a steam boiler used in a 55 MW coal-fired thermal power plant based on hot air temperature. The results show the effect of hot air temperature on the unit product cost of a boiler, unit product cost of the air preheater, and exergetic efficiency of the boiler system. Finally, the optimization has been done for the unit product cost of air preheater and the unit product cost of the boiler regarding the hot air temperature. Vuckovic et al. (2014) have performed the advanced exergy analysis and exergoeconomic evaluation of the thermal processes in an existing industrial plant.
Elmzughi et al. (2020a) conducted thermoeconomic analyses for a simple typical thermal power plant, where they employed the specific exergy costing approach and sensitivity cost analysis. Regarding their basic data, the potential improvement has been found to be maximum in the boiler. The exergoeconomic factor for the boiler has been 0.23, and the total cost of the plant has been 14,000 $/hr. Radwan and Bahoor (2020) presented a detailed thermoeconomic study for a hypothetical cogeneration power plant cycle, where they analyzed the performance of the cycle including thermodynamic and exergoeconomic view points. They determined the advanced parameters related to energy utilization.
Elmzughi et al. (2020b) worked on a power plant cycle with a conventional steam Rankine cycle with high, intermediate, and low-pressure turbines, four low-pressure heaters, and two high-pressure heaters. The studied boiler temperature, condenser pressure, and load percentage are set to be variables. The exergetic efficiency, exergy destruction, improvement potential, and exergy are determined. The total irreversibility decreases with increase in the boiler temperature, and as the load increases by 1 %, the total irreversibility rise by 4.5 MW, with a maximum value of 440 MW. The maximum improvement potential takes place in the steam generator, reheater, and condenser, with 163.0, 26.0, and 8.0 MW, respectively. The lowest exergetic efficiency is 9.2 % for the condenser, while the highest is for the deaerator with 97%.
In the present paper, the first and second laws of thermodynamics with the economic models are applied to identify the performance of the critical cycle components and the potential for exergy efficiency improvements for an industrial energy supply plant. Here, the desired cogeneration thermal power cycle is to be introduced and thermally and economically analyzed. The methodology is based on the Theory of Exegetic cost. Thermodynamic properties of the inlet and outlet points of each component in the steam plant have been specified via the Thermax program, and economic analyses by employing both Excel and Matlab software packages.
The case study represents a cogeneration thermal power cycle case, with a total power capacity of 350 MW, with a conventional steam Rankine cycle with high, intermediate, and low-pressure turbines, four low-pressure heaters, and two high-pressure heaters, as indicated in Figure 1. Thermodynamics properties of the inlet and outlet points are obtained from Thermax and Matlab software packages.
The nominal values of the design parameters are well defined in Table 1. To conduct the energy, exergy, exergoeconomic, and sensitivity analyses, the main parameters associated with this study are listed in Table 2. Detailed mathematical models are presented. These include thermodynamic and thermoeconomic mathematical models for the working fluid processes.
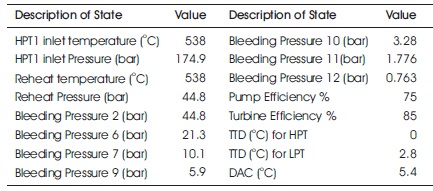
Table 1. Design Parameters of the 350 MW Steam Power Unit
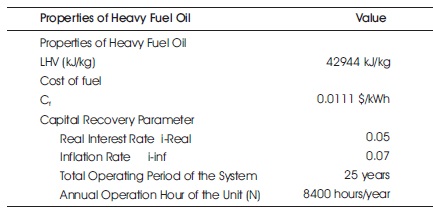
Table 2. Different Parameters Used in the Calculations
Most of the analytical analysis for the desired power cycle, concerning mass, energy, and exergy balances, was introduced (Elmzughi et al., 2020a, 2020b; Radwan & Bahoor, 2020). Here, the main mathematical relations should be briefly presented for the steady-state steady flow process and the kinetic and potential energy changes are negligible.
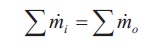
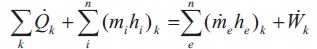
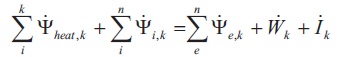
The exergy transfer by heat is given by;
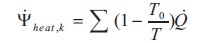
The specific exergy is given by;

The exergy rate is;
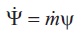
Exergy balance for a single component is given by;

The exergetic efficiency can be defined for each component as follows;
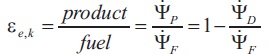
The exergetic efficiency of the power cycle is given as;
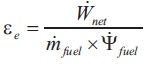
The ratio of simplified exergy is defined as follows (Elmzughi et al., 2020a, 2020b; Radwan & Bahoor, 2020).
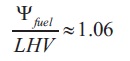
The improvement potential is given by the following expression (Elmzughi et al., 2020a, 2020b; Radwan & Bahoor, 2020).
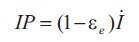
According to the specific exergy costing method, the cost balance equation for a given component can be written as (Elmzughi et al., 2020a, 2020b; Radwan & Bahoor, 2020),
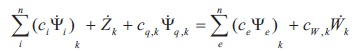
The annualized equipment cost is given by;

where (PEC) is the equipment purchasing cost and CRF is k the capital recovery factor given by:
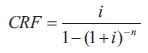
Here, the effective interest rate is given by;
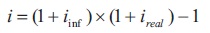
The capital cost rate ($/hr) can be written as;
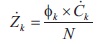
The factor φk=1.06 takes into account the maintenance cost and N annual operation hours of the plant. Next, the specific exergy cost is defined as;
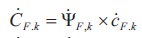
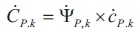
Table 3 presents the assumed economical models to estimate the purchase cost for different cycle components (Baghernejad & Yaghoubi, 2011; Bejan et al., 1996; Elmzughi et al., 2020a, 2020b; Radwan & Bahoor, 2020; Silveira & Tuna, 2003; Uche et al., 2001).
The cost rate of the destructed exergy within the component k, can be found as (Xiong et al., 2012);

The single exergoeconomic factor is defined by the equation below for the k unit of the system (Bejan et al., 1996; Xiong et al., 2012) as;
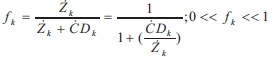
For the whole plant, the exergoeconomic factor is:
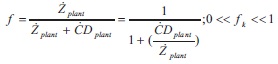
To solve the cost balance equations for each component, the number of unknown cost parameters is higher than the number of the cost balance equations for that component. Auxiliary exergoeconomic equations should be developed to solve this problem as follows;
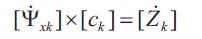
where [Ψxk ],[ck ] and [Zk ] are the matrices of the exergy rate, exergetic cost vector, and vector of Z economic factors, k respectively.
In general, auxiliary equations must be formulated based on the F–P rules given by Elsafi (2015) as the number of streams are more than devices. A system of equations can, therefore, be developed by formulating the costing equations for each component along with the auxiliary equations. Applying a theory of energetic cost for every component, the system is given as follows:
Steam generator,
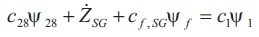
Reheater (RH),
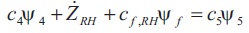
High-Pressure Turbine (HPT),
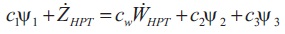
Intermediate Pressure Turbine (IPT),
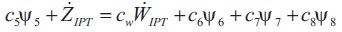
Low-Pressure Turbine (LPT),
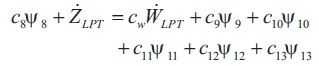
Condensate Extraction Pump (CEP),
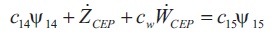
Boiler Feed Pump (BEP),

Condenser (COND),
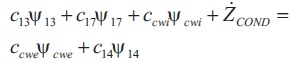
First Low-Pressure Heater 1 (LPH1),
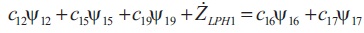
Second Low-Pressure Heater 2 (LPH2),
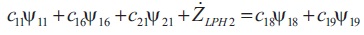
Third Low-Pressure Heater 3 (LPH3),
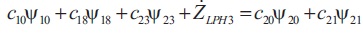
Fourth Low-Pressure Heater 4 (LPH4),

Deaerator (DEA),
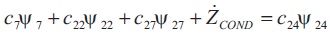
First High-Pressure Heater 1 (HPH1),
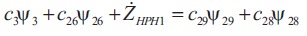
Second High-Pressure Heater 2 (HPH2),
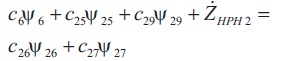
For the system of Equations (23-37) to be solved, additional auxiliary equations should be formulated.
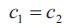

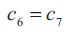
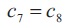
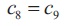

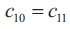
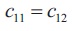
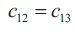
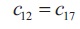
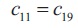
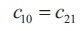
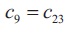
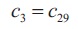
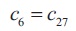

Now, the mentioned parameters could be calculated, including the exergetic efficiency, rate of exergy destruction, cost rates associated with the capital investment, operating and maintenance expenses, the exergy destruction cost rate, the relative cost difference, and the factor, where the thermodynamic properties of the inlet and outlet points of each component in the steam plant have been specified via Thermax program.
The calculations are made while the environmental 0 reference temperature and pressure are taken as 24 C and 1 bar, respectively. The procedure details are presented by Bejan et al. (1996), Elmzughi et al. (2020a, 2020b), Lazzaretto and Andreatta, (1995), Radwan and Bahoor (2020), Vuckovic et al. (2014). The plant is considered to be operated for 8400 hours annually, while the real interest rate is 0.05, with an inflation rate of 0.07, and the lifetime of the system is 25 years.
In Figures 2 and 3, the exergy destruction and improvement potential for each component of the thermal power cycle are presented, respectively. The maximum exergy destruction and improvement potential occurred in the steam generator with values of 75%, and 81%, respectively. This confirms that the steam boiler dominates the energy movement in the considered power cycle, where it is the component with priority for any desired plant upgrading program. This could include the use of an alternative fuel type, better combustion equipment, high- quality control operating program, and installing a thermal waste recovery system associated with the exhaust gases.
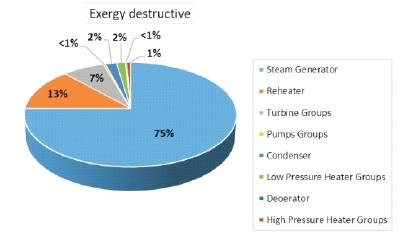
Figure 2. Exergy Destruction of Each Component of the Power Plant in Percent
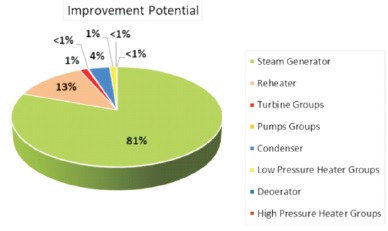
Figure 3. Improvement Potential of Each Component of the Power Plant in Percent
Figures 4 and 5 represent the exergoeconomic factor and total cost for each cycle component. Here, the condensate extraction pump comes with the maximum exergoeconomic factor followed by the deaerator and intermediate pressure turbine, while the steam generator has the maximum total cost followed by the reheater and low-pressure turbine. The minimum exergoeconomic factor and minimum total cost are for the low-pressure heater 1 and condensate extraction pump, respectively.
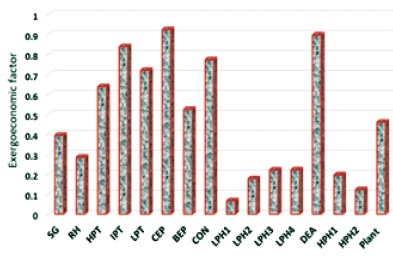
Figure 4. The Exergoeconomic Factor of Each Component
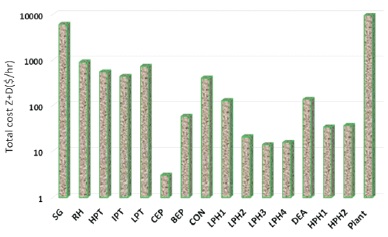
Figure 5. The Total Cost of Each Component
The consequences of the expected span of the plant lifetime; 20, 25, and 30 years, on the exergoeconomic factor and total cost of each cycle component are considered in Figures 6 and 7, respectively. For plant lifetime over 20 years, the effects seem to be relatively not that much, where both the exergoeconomic factor and total cost mildly decrease with the five-year step in the plant lifetime. The effect on the exergoeconomic factor is not relatively uniform for all cycle components, while the effect on the total cost seems to be relatively uniform for all cycle components, noticing the different purchase cost models.

Figure 6. Effect of the Plant Lifetime on the Exergoeconomic Factor of Each Component
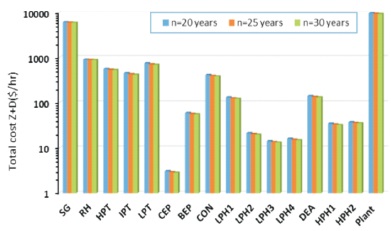
Figure 7. Effect of the Plant Lifetime on the Total Cost of each Component
The influences of the boiler temperature on the unit cost of steam and work are shown in Figures 8 and 9. For plant lifetime of 25 years, and for the increase of the boiler temperature from 450 to 800 ºC, the unit costs of steam and work increases from 0.028 to 0.039 $/kWh, and from 0.0136 to 0.0164 $/kWh, respectively. Here it is clear that both curves for 25 and 35 years almost coincide for the variation of the steam cost, while it varies with the cost of the work.
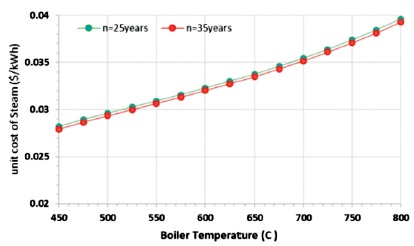
Figure 8. Effect of the Boiler Steam Temperature on the Unit Cost of Steam

Figure 9. Effect of the Boiler Steam Temperature on the Unit Cost of Work
Comparing with Elmzughi et al. (2020), for the simple power cycle under the same conditions and boiler temperature range, the unit costs of steam and work vary from 0.031 to 0.0277 $/kWh, and from 0.0394 to 0.0348 $/kWh, respectively. Here, the steam unit cost is mostly the same for both power cycles, while the work unit for the simple cycle costs more than double the value. It seems to have the same arrangements for the fuel type and fuel system, identical boiler design, and operating program, while the turbine arrangements are different for both cases due to the presence of the heaters.
In this paper, a cogeneration steam power plant is studied, where thermoeconomic analyses have been done using employ Thermax and Matlab computer software packages. The major destruction occurred in the steam generator with 75% of the total cycle. The maximum improvement potential has been found to be 81% for the steam boiler. This confirms the priority for any upgrading program leading to a high percentage plant performance improvement. This could include the use of an alternative fuel type, better combustion equipment, high-quality control operating program, and installing a thermal waste recovery system associated with the exhaust gases. The exergetic efficiency is 66.21% for the whole powerplant cycle.
The exergoeconomic factor and the total cost are found to be 0.45 and 9249 $/hr, respectively. The unit cost of steam, work, and cooling water for the plant were found to be 0.030, 0.014, and 0.45 $/kWh, respectively. The highest exergoeconomic factor has been measured in the turbine group, followed respectively by the boiler and condenser drain pump. For plant lifetime over 20 years, the lifetime effects on the exergoeconomic factor and total cost seem to be relatively negligible. The steam unit cost is mostly the same for the simple and cogeneration power cycles, under the same conditions, while the work unit for the simple cycle costs more than double. Because of the great achievements in energy efficiency and total plant cost needed, simple cycles should be updated properly.
To develop more accurate models and obtain results that can be applied to the thermal power plant, the following recommendation should be taken into consideration.