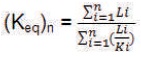
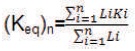
where L is the thickness of the i^th layer in the layered system, K_i is the coefficient of permeability of that layer and K_eq is the equivalent permeability of the deposit.

In this study, a comprehensive evaluation has been done to compare the values of experimental and theoretical permeability by considering a number of combinations of soils having varying specifications and properties. Here, the behaviour of two layers and three layers stratified deposits consisting of sand, silt, and clay subjected to perpendicular flow with respect to bedding plane has been presented. Also the factors and mechanism affecting the permeability of such layered deposits has been briefly described in subsequent sections so as to have a good understanding of such flow conditions.

A number of studies have been carried out both in India and around the world on this topic. Prof. A. Sridharan and Prakash of IISc Bangalore has worked on two and threelayered soils subjected to a flow normal to the bedding plane (Sridharan & Prakash, 2002; Prakash & Sridharan, 2013). From these studies, they concluded that observed equivalent permeability is quite different from what they actually calculated using Darcy's law. They stated that this variation is primarily because of interaction among the materials of the stratified deposit.

Considering the influence of permeability on the overall soil behaviour, extensive research has been carried out in this field for working out the the mechanism and factors influencing soil behaviour under varying soil formations and conditions.

Uppot and Stephenson (1989) explained how organic and inorganic permeants were made to flow through two clays with differing mineral composition and the changes in permeability patterns were studied due to the reaction between the clay minerals and the permeants. It was concluded from this study that permeability typically depends on the chemical composition of soil and that of permeant, also the test procedure employed had a significant bearing on the results.

Sridharan and Prakash (2002) researched on a two-layered soil-system used for comparing the experimental and theoretical coefficient of permeability values. A detailed analysis revealed that the mutual interaction between layers of soil system significantly influences the equivalent permeability of the soil system. The value of theoretical permeability calculated using Darcy's law has a considerable variation when compared to the experimentally calculated coefficient of permeability. Also, the deciding factor is the relative position of constituent layers, i.e., the permeability of exit layer controls whether the experimental value is greater or less than the theoretical value of permeability of the soil system. After conducting multiple tests, it was concluded that the individual layer thickness and their permeability, positioning of layers and the direction of fluid flow i.e., whether parallel or perpendicular, were the factors governing coefficient of permeability of the layered soil system.

de Brito Galvão, et al. (2004) studied the influence of mutual interaction on the coefficient of permeability of different mixtures by using soils of varying physical and chemical properties. Upon the addition of lime to saprolitic soil, the coefficient of permeability increases upto five times when lime was around two percent and any further addition resulted in a net decrease of permeability. In case of lateritic soil the coefficient of permeability decreased upon lime addition. These changes in both the soils are directly linked to the strength of chemical bonds and aggregation of particles, i.e., stronger bonds in saprolitic mix as compared to lateritic mix.

Prakash and Sridharan (2013) used a three-layered soilsystem for comparing the experimental and theoretical coefficient of permeability values. After the comparative analysis, it was concluded that the permeability of bottom layer is the primary parameter which decides whether the calculated theoretical value is greater or lesser than the experimentally calculated equivalent permeability value. It can be inferred from this observation that the equivalent coefficient of permeability depends not only on the individual permeability values of constituent layers but also on the relative positions of constituent layers of the soil deposit.

Yanful et al. (1990) formed a prototype liner of sodium bentonite and Ottawa sand, and these materials were properly mixed at a definite moisture content and reinforced into wooden frames. Later permeability tests were conducted on it in the laboratory. The back-pressure saturated triaxial permeameter tests were conducted on undisturbed and remoulded samples and results were verified with the on-field permeability tests.

Alam et al. (2015) used a two layered soil system and the influence of the exit layer permeability on the overall permeability of system was evaluated. After evaluation it was concluded that, when the exit layer is less pervious than the top layer, overall permeability of deposit is more than the exit layer permeability. When the permeability of exit layer is more than top layer, the overall permeability of deposit is less than isolated permeability of exit layer. This variation in permeability was attributed to the effect of interface between the two soil layers. This study also questioned the validity of using Darcy's law for evaluating permeability of such layered deposits as this law does not take into account the influence of the interface on overall permeability.

For this experimental study, samples consisting of sand, silt, and clay where collected from different locations in Kashmir valley. The sand was collected from Sindh banks near Duderhama Ganderbal, silt was collected from Nishat Srinagar and clay from Rangil Ganderbal. All the collected samples where oven dried for 24 hours at 105 ^oC-110 ^oC temperature. All these samples were then subjected to particle size distribution analysis which included both sieving and hydrometer analysis (Figure 1, 2 & 3).

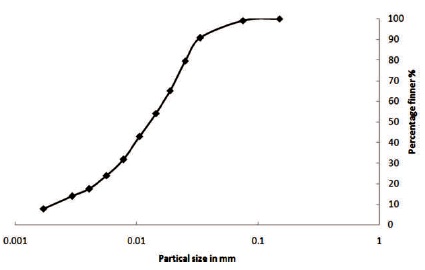
Figure 1. Particle Size Distribution of Clay

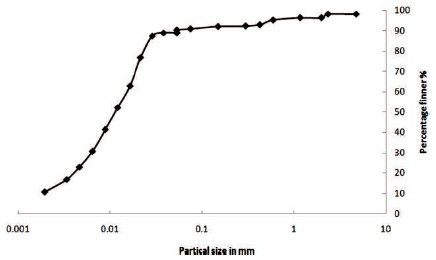
Figure 2. Particle Size Distribution of Silt

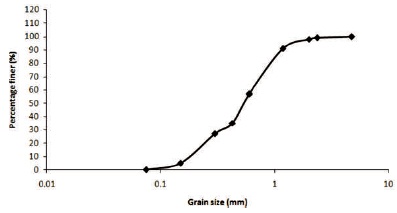
Figure 3. Particle Size Distribution of Sand

Then density bottle method was used for calculating specific gravity of sand, silt, and clay in accordance with the procedures mentioned in Bureau of Indian Standards (1980). The specific gravity for clay, sand, and silt was found to be 2.27, 2.58 and 2.54 respectively. For the present study, Constant head permeameter was used for determining the permeability of sand and falling head permeameter was used for determining the permeability of clay and silt. The tests were carried out at optimum moisture content corresponding to maximum dry density. The above-mentioned materials were used for two layer and three-layer configurations and the coefficient of permeability was determined both theoretically and experimentally for different cases of above-mentioned configurations. Both for two layers and three-layer system a total of twelve cases were tested. Each mould was filled with different layers and each layer was compacted up to the maximum dry density. After preparing the mould water was passed through it so as to have complete saturation of the layered soil, then standpipe was connected to the mould and the fall in the water levels of standpipe was noted after regular intervals of time (Falling Head Method from Equation (2). During this experiment, the temperature was also recorded. In this way, the permeability of clay, silt, and sand was determined.

(3)

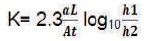
3. Results and Discussions

3.1 For Two Layer System

With different combinations of the sand, silt and clay six twolayered setups were created (Figure 4) and these six can broadly be categorized into two groups:

• Those where the exit layer is less pervious than the inlet layer.
• Those where the exit layer is more pervious than the inlet layer.

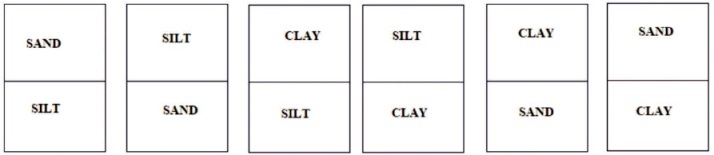
Figure 4. Six Combinations of Double Layered Soil System

After conducting permeability tests it was found that when the exit layer is less pervious the experimental coefficient of permeability was less than that calculated by Darcy's law. On the other hand, the coefficient of permeability calculated from Darcy's law was more than the experimental one when exit layer was more pervious. It can now be concluded that when the flow is normal to the bedding plane the experimental coefficient of permeability may be more or less than the theoretical coefficient of permeability. And it primarily depends on whether the exit layer is more or less pervious than the inlet layer respectively.

Consider two layers forming a layered deposit with permeability coefficients as k_in and k_out.

Case 1 (k_out < k_in):

At the interface of two layers the flow rate is decelerated because of lesser permeability of exit layer. From the experimental data (Table 1), it seems that coefficient of permeability of layers of the stratified deposit is different from the individual values of the layers when considered individually. This is due to the continuous flow over the entire thickness of the layered soil with the result k_(measured) < k_(calculated).

Case 2 (k_out > k_in):

The higher value of permeability of the exit layer creates a suction which in turn increases the flow rate through the inlet layer for maintenance of continuity. As mentioned in case 1 the values of k_in and k_out in the stratified deposit are quite different from the values of coefficient of permeability of same layers when considered individually. Consequently K_(measured) > K_(calculated). Therefore it can be said that permeability of the exit layer controls the measured permeability in a stratified deposit (Table 1).

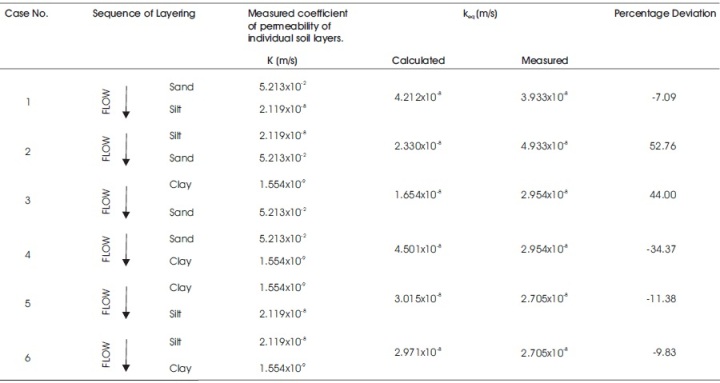
Table 1. Results from the Experiments on Two-Layer Systems

3.2 For Three Layer System

In the case of the three-layer system, a similar pattern like that in the two-layer system is observed, that is the exit layer controls the measured equivalent coefficient of permeability of a stratified deposit. Here also six threelayered systems were considered and the layer below in the direction of flow acts as exit layer (Figure 5). The variation in the measured and calculated values depends on the mutual interaction amongst the layers. In the following section, each case has been qualitatively analyzed and some inferences have been made.

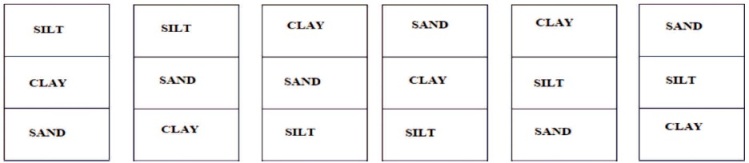
Figure 5. Six Combinations of Three Layered Soil System

Case (1): In this case, the sand layer forms the exit layer followed by clay and silt upper-side. There is a possibility of exit layer increasing the permeability of overlying layers. The examination of the individual values of k of clay and silt layers is given in Table 2 and it can be seen that the permeability of clay layer may not have much influence on the permeability of silt layer. Thus, the measured value of k_eq is likely to be more than that calculated.

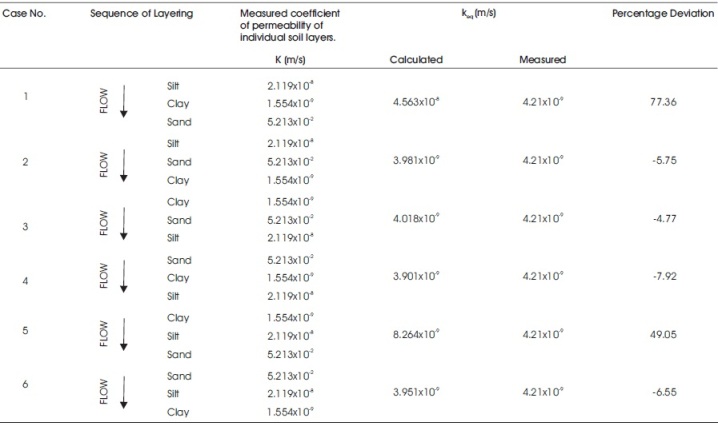
Table 2. Results from the Experiments on Three-Layer Systems

Case (2): Here the exit layer is of clay, sand is the middle layer while the silt forms the top layer. In this case, also the permeability of each layer is dependent on the mutual interaction between the layers. The clay layer reduces the permeability of the sand layer and this decrease negatively impacts the expected increase of permeability of silt at top. Therefore we see the measured value of k_eq is less than the calculated value.

Case (3): Here silt, sand, and clay form the bottom-most, middle and topmost layers respectively. This case follows the similar pattern as that of case 2, however, the extent of reduction of permeability of sand by silt layer is lesser which in turn influences expected increase in permeability of clay layer by the sand layer, here also the measured value of k_eq is likely to be less than that calculated.

Case (4): In this case, the silt forms the exit layer while as the clay and sand form the middle and top layers respectively. Here we do not see a considerable increase in permeability of clay as silt itself has less permeability. However, any increase tends to reduce the permeability of sand above. Hence, the measured k_eq is expected to be less than the calculated value.

Case (5): In this case, layers are arranged in the increasing order i.e., clay at the top, followed by silt and sand at the bottom. The exit layer here has the maximum value of permeability. Hence, this is a clear case where the measured value of k_eq has to be more than calculated value.

Case (6): Here, the sand layer is at the bottom followed by clay and silt upwards. Sand layer is expected to increase the permeability of clay placed above. However from Table 2 it can be noted that clay does not influence the permeability of silt layer above it, i.e., very less decrease in k of silt by clay. Thus, the measured value of k_eq is likely to be more than that calculated.

Conclusion

In this study, a comparison has been made between observed and theoretical values of permeability of 2- layered and 3-layered stratified soil deposits. The experimental results confirm that the value of k (measured) directly depends on the positioning of different layers and it is very difficult to predict deviation of measured k value from calculated value because of the complex interactions amongst materials of layers. The coefficient of permeability of the exit layer has a profound influence on measured permeability and it governs the variation between measured and calculated values of permeability. However, exit layer permeability is not the sole parameter but this variation also depends on the length of individual layers of the deposit.

References

[1]. Alam, J., Muzzammil, M., Singh, H. P., & Gupta, P. (2015). Permeability of stratified soils for flow normal to bedding plane. Aquatic Procedia, 4, 660-667.

[2]. Bowles, J. E. (2001). Foundation Analysis and Design, McGraw-Hill.

[3]. Bureau of Indian Standard (1980). Methods of test for soils, Part III: Determination of specific gravity, Section 2: Fine, medium and coarse grained soils (Standard No. IS 2720).

[4]. de Brito Galvão, T. C., Elsharief, A., & Simões, G. F. (2004). Effects of lime on permeability and compressibility of two tropical residual soils. Journal of Environmental Engineering, 130(8), 881-885.

[5]. Hamidon, A. (1994). Some laboratory studies of anisotropy of permeability of kaolin (Doctoral dissertation), University of Glasgow.

[6]. Nagy, L., Tabácks, A., Huszák, T., & Varda, G. (2013). Comparison of permeability testing methods. In Proceedings of the 18th International Conference on Soil Mechanics and Geotechnical Engineering, (Vol. 1, pp. 399-402). Paris.

[7]. Prakash, K., & Sridharan, A. (2013). Permeability of layered soils: An extended study. Geotechnical and Geological Engineering, 31(5), 1639-1644.

[8]. Ranjan, G., & Rao, A. S. R. (2007). Basic and applied soil mechanics. New Age International.

[9]. Sridharan, A., & Prakash, K. (2002). Permeability of twolayer soils. Geotechnical Testing Journal, 25(4), 443-448.

[10]. Uppot, J. O., & Stephenson, R. W. (1989). Permeability of clays under organic permeants. Journal of Geotechnical Engineering, 115(1), 115-131.

[11]. Yanful, E. K., Haug, M. D., & Wong, L. C. (1990). The impact of synthetic leachate on the hydraulic conductivity of a smectitic till underlying a landfill near Saskatoon, Saskatchewan. Canadian Geotechnical Journal, 27(4), 507-519.