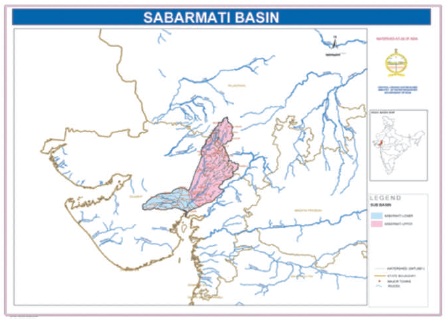
Figure 1. Location Map of Sabarmati River Basin (Source: http://cgwb.gov.in/watershed/Maps/SABARMATI.jpg)
Rainfall is the most important and an essential natural source of water, which is utilized for different purposes. Hydrological analysis and modelling becomes very popular in the field of water resources management. Rain gauge is a common instrument that is used to measure the amount of rainfall and it also provides the rainfall distribution pattern. These data prove to be very useful and can be used for different purposes, such as design of hydraulic structures, flood control, irrigation canal design, etc. Thus, rainfall data are prime input parameter and it is most critical for a river basin to have an optimum number of rain gauges in catchment area of the river. This paper has basically analyzed whether the Sabarmati River basin has an optimum number of rain gauges or not. If not then design of optimum number of rain gauge is an important task in the river basin. Thus, according to IS:4987-1994, Optimum number of rain gauges in Sabarmati river basin is 37, while existing rain gauges are 31. So, Additional 6 rain gauges are required in basin and their location is also proposed in this paper based on Thiessen polygon method, which is used for equal areal rainfall distribution in the basin.
Rainfall is one of the most common form of precipitations. The intensity of rainfall varies with place and time. The variation in intensity of rainfall is responsible for many water problems like flood and drought. Rainfall data provide essential input for effective planning, designing, operating, and managing of water resources projects (Adhikary et al., 2015). Rainfall data are the most important data in the field of water resources engineering, which are employed in various analysis, i.e., water budget analysis, flood forecasting analysis, flood mitigation management, stream flow measurement, construction of any hydraulic structures, etc. (Cheng et al., 2008). Direct measurement of rainfall can only be achieved by rain-gauges, and rain gauge networks are often installed to provide measurements that characterize the temporal and spatial variations of rainfall (Putthividhya & Tanaka, 2012). An optimum number of rain gauge network in any river basin can provide immediate, precise, and accurate data of rainfall, which is very important for design of hydraulic structures and also important in flood control management (Patel et al., 2016). Location of rain gauge station is affected by many factors, i.e. accessibility, easiness of maintenance, topographical aspects, etc. In addition to this, the minimum density of a rain-gauge network is dependent on the time resolution (or scale) of the desired rainfall measurements. Therefore, a methodology for performance evaluation and augmentation of an existing rain-gauge network is crucial, in that it can help to understand the capability of an existing network and the quality of the data it provides (Sen, 2013). Precipitation uncertainty greatly affects hydrologic model flow simulations and predictions, and in a real-time flood forecasting and warning situation, the selection of a proper gauge network becomes an important issue (Tsintikidis et al., 2002). If the river basin has less number of rain gauge compared to the areal extent of a storm, it is important to have more number of rain gauges for equal and accurate distribution of rainfall to get a perfect representative picture of the storm over a catchment (Al-Aboodi & Al-Abadi, 2014).
The aim of the optimum rain-gauge network design is to obtain data that define statistical distribution of the meteorological elements with enough accuracy for practical situations.
The Sabarmati River basin is taken as the study for analysis of optimum number of rain gauge network (Figure 1). The Sabarmati basin has a total catchment area of 21674 sq. km. Out of which, 4124 sq. km lies in Rajasthan State and the remaining 18550 sq. km lies in Gujarat. In this paper, analysis is done only for Gujarat state river basin, which covers an area of 18550 sq. km. The major tributaries are Wakal, Sei, Shedhi, Harnav, Guhai, Hathmati, Khari, Meshwo, Mazam, and Mohar.

Figure 1. Location Map of Sabarmati River Basin (Source: http://cgwb.gov.in/watershed/Maps/SABARMATI.jpg)
Sabarmati River originating in Rajasthan flows generally in South-West direction. It enters in the Gujarat State and passes through the plains and continues to flow in the same direction and joins the Gulf of Cambay in Arabian Sea. Total length of Sabarmati river is 371 km from which 48 km is in Rajasthan and 323 km is in Gujarat.
Rainfall data have been collected from State Water Data Center in Gujarat. Rainfall data of last 15 years have been collected for a total 31 Rain Gauge stations, which are located in the Sabarmati River basin. For analysis of rainfall pattern rainfall, data from 2000 – 2015 were selected.
There are a total of 31 rain gauge stations in Sabarmati River basin in Gujarat State covering 18550 sq. km area. With the help of latitude and longitude all rain gauge stations are located on the Google map. Location of rain gauge station is given in Figure 2.
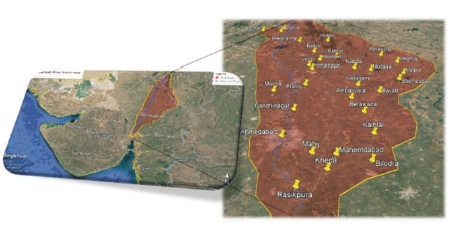
Figure 2. Location of Rain Gauge Stations in Sabarmati River Basin
Rainfall data have been collected and analyzed based on arithmetic mean average method. Rainfall data of the last 15 years from 2000-2015 was analyzed and an average annual rainfall of a particular station has been carried out. Average annual rainfall for each station, their tributary, and district of all stations are given in Table 1.
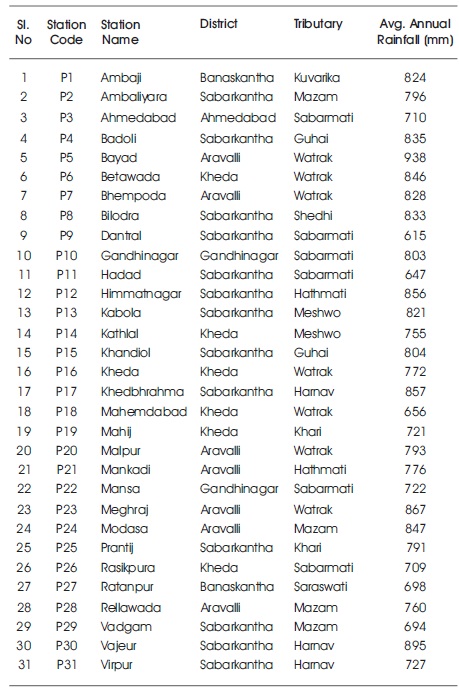
Table 1. Rain Gauge Station Details and their Location
Maximum average annual rainfall is noted at rain gauge station Bayad and minimum average rainfall is noted at Dantral rain gauge station from Figure 3. Total average annual rainfall of Sabarmati River basin is 781 mm for last 15 years.
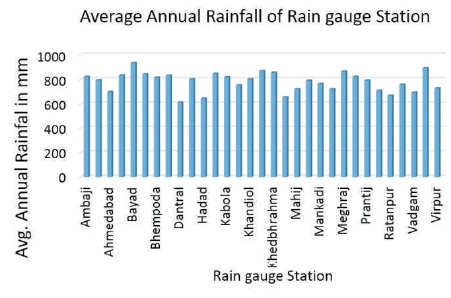
Figure 3. Average Annual Rainfall Analysis of Rain Gauge Station for 15 year
From Figure 4, it is clear that maximum average annual rainfall is noted in the year 2006 (1453 mm) and minimum average rainfall is noted in the year 2002 (350 mm). A graphical representation is given in Figure 4 for yearly average annual rainfall of Sabarmati River basin from 2000 to 2015.
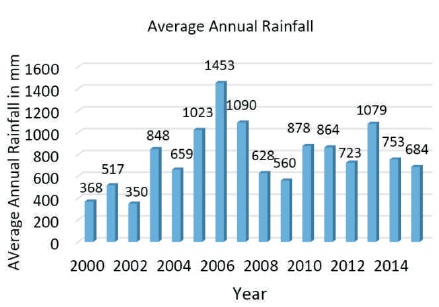
Figure 4. Yearly Average Annual Rainfall Analysis for Sabarmati River Basin
Optimum number of rain gauges can be calculated based on Indian Standards (IS: 4987:1994). Based on IS: 4987:1994, clause-3.2 it is given that for plain area of river basin, one rain gauge station is required for 500 sq. km area. Sabarmati River basin have a plain catchment area throughout the basin.
Optimum number of rain gauge required:-
So, based on IS: 4987:1994, optimum number of rain gauge required is 37 for catchment area of 18,550 sq. km, which is for Sabarmati River Gujarat basin.
IS: 4987:1994 has also given a procedure for optimum number of rain gauge network design. The aim of optimum network design is that, by interpolation between values of different stations, it should be possible to determine with sufficient accuracy, for practical purposes, the characteristics of the basic hydrometeorological elements at any point of interest. By the characteristics meant, all quantitative data, averages and extremes that define the statistical distribution of the hydrometeorological elements are studied. Following are the steps for designing.
Step 1: Calculate the total of annual average rainfall of all rain gauge stations
T = P1+P2+P3+………..+P31 = 24,194 mm
Step 2: Calculate the mean rainfall in the catchment
M = T÷ 31 = 24,194 ÷ 31 = 781 mm
Step 3: Calculate the sum of the square of the rainfall of these 31 rain gauges as,
SS = (P1)2 + (P2)2 +……..+ (P31)2 = 19056997.26
Step 4: Calculate as follows,
T2 ÷ 31 = 585351451/31 = 18882304.86
Step 5: Calculate the variance S (square of standard deviation)
S2 = {SS – (T2 ÷ 31)}/ (31-1)
S2 = {19056997.26 - 18882304.86} ÷ 30
S = 76.30
Step 6: Calculate the coefficient of variance Cv
Cv = {100 × S} ÷ M = 9.77
Step 7: Calculate the allowable percentage error E
N= Cv ÷ E2
N= Optimum number of rain gauge = 37
E= 1.60%
Optimum number of rain gauge is 37 if the allowable percentage error should be 1.6%.
As per above calculation, optimum number of rain gauges in Sabarmati River basin required is 37. So, additional 6 rain gauges are required in Sabarmati River basin in Gujarat State. These six rain gauges should be located in the basin such that rainfall is equitably distributed within the basin.
The additional six rain gauges should be located as additional rain gauge stations, which depends upon the spatial distribution of existing rain gauge stations, and the variability of rainfall over the basins. With the limitations of accessibility to new sites and facilities available for maintaining these stations, additional rain gauges should be located so as to have an even distribution over the entire basin. Six new rain gauges are proposed in Sabarmati River basin, which are Vijapur, Ranasan, Karoli, Talod, Aroda, and Kajipur. These rain gauges are evenly distributed in the basin so that rainfall is also equally distributed to all the rain gauge stations.
The Thiessen Polygon method is the most approximate method since rainfall varies in intensity and duration from place to place. Hence rainfall recorded by each rain gauge station should be weighed according to the area it is assumed to represent. Figure 5 shows a Thiessen network constructed for Sabarmati River basin. The polygon formed by the perpendicular bisectors around a station encloses an area which is everywhere closer to that station than to any other station.
It is concluded from the paper that existing rain gauge stations in Sabarmati river basin are not optimum. As per calculation based on IS: 4987 1994, optimum number of rain gauge stations required in Sabarmati River basin is 37. So, additional 6 rain gauge stations are required to be installed in the river basin. These additional six rain gauges are Vijapur, Ranasan, Karoli, Talod, Aroda, and Kajipur. The final rain gauge network is shown in Figure 5. The rain gauge network is in such a way that all rainfall is equally and evenly distributed within the river basin. So, Thiessen polygon method is the best suitable method for this analysis.
Thiessen polygon network for new rain gauge station is prepared for the Sabarmati river basin given in Figure 6. It is also concluded from a calculation that rain gauge station having maximum allowable error is 1.6%.
The author is thankful to State Water Data Center (SWDC), Gandhinagar for providing necessary data to carry out this study. He is also thankful to Hardik Chaudhari, Akash Gupta, and Dhruv Patel, students of Department of Civil Engineering, Nirma University for their help in this study.