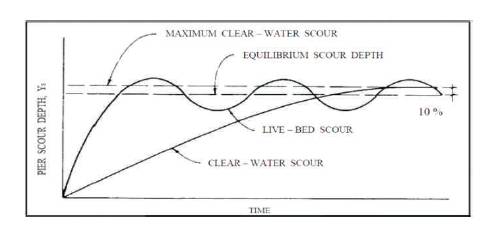
Figure 1. Pier Scour Depth in a Sand-bed Stream as a Function of Time.(Raudkivi and Ettema 1983)
When an alluvial stream is partially/fully obstructed by a bridge pier, scour takes place in the vicinity of the pier. Estimation of this scour depth is a major concern of bridge engineers. The underestimation of scour depth leads to unsafe design, while an overestimation leads to uneconomical design. Therefore, estimation of anticipated maximum scour depth for given design discharge is essential. Scour at bridge piers is mainly affected by pier, flow and sediment characteristics. On the basis of field investigations, many investigators have suggested criteria for assessing maximum scour depth around bridge pier in alluvial bed. These studies are mainly regarding scour around bridge piers at normal flow conditions. Adequate information in connection with scour at bridge pier at different depth of flow, piers size and sediment size is not available. From the observation, we found that scour depth goes on increasing with increase in pier diameter.Out of these, the effects of pier size and shape, and of the approach flow angle on scour depth are fairly well understood. The effects of flow depth and sediment non-uniformity on scour depth have been also studied in detail. In this paper, a method has been presented to estimate the time variation of scour depth with uniform and nonuniform sediments. The procedure has been developed for computing the time variation of scour depth which is based on the dimensional analysis, and considering the horse-shoe vortex system to be the main scouring agent.
The review of literature had revealed that most of the existing approaches for describing the temporal variation of scour depth near a pier is an important aspect. It becomes pertinent to some laboratory and field cases for which the time factor plays a significant role. Important investigations on the time variation of scour have previously been carried out by Chabert and Engeldinger (1956), Ettema (1980), Kothyari (1990), Yanmaz et al. (1991), Melville and Coleman (2000) and others.
The studies that has been carried by the different investigators is mainly based on the dimensional analysis of different parameters describing the scour process by regression analysis of laboratory and field data, and thus giving the equation by drawing an envelope curve, and this results in overestimation. But majority of the investigators have used the dimensional analysis approach.
The process of local scour can occur in one of the two ways: (i) clear-water scour; and (ii) live bed scour. Clearwater scour occurs when the bed material upstream of the scour area is at rest i.e. there is no sediment supply into the scour hole by the upstream flow. The bed shear stresses away from the scour area are thus equal to or less than the critical or threshold shear stress for the initiation of particle movement. Live-bed scour, also referred to as scour with sediment transport, occurs when there is general bed load transport by the stream i.e. in this type of scour process, the sediment carried by the upstream flow is continuously supplied into the scour hole.
From Figure 1, it can be observed that the scour depth is variable with time, and it increases with increase in time. Also clear-water scour is higher than live-bed scour. Hence in present case, temporal variation of clear-water scour at cylindrical bridge pier has been studied.

Figure 1. Pier Scour Depth in a Sand-bed Stream as a Function of Time.(Raudkivi and Ettema 1983)
Thus in this paper, mainly prediction of temporal variation of clear-water local scour at bridge piers based on dimensional analysis of parameters describing scour depth has been discussed. Variation of the maximum scour depth for the laboratory data for clear-water including 100 hours data of different investigators with the parameter, involving size of the piers, flow and sediment characteristics has been presented.
The mechanism of local scour is a very complex threedimensional separation due to large variations in the flow field conditions from one site to another, moreover, numerous variables describing the flow, the fluid, the sediment characteristics, the channel and the obstruction geometry etc. The mechanism associated with the problem of local scouring have been studied for more than six decades. The investigators have pointed out that the vortex systems are the basic mechanism of local scouring, since many experimental works exist on the problem of local scouring. However, in most of these studies, emphasis has been on the formulation of scour depth equations, rather than modelling the problem of local scouring. A summary of important experiments on local scouring has been given by Chabert and Engeldinger (1956), Melville (1975), Breusers et al. (1977),Melville and Raudkivi (1977), Dargahi (1982), Chang (1988), Dey (1997), Graf and Istiarto (2002) and others.
The basic mechanism causing local scour at piers is the down-flow at the upstream face of the pier and formation of vortices at the base. The flow decelerates as it approaches the pier coming to rest at the pier face. The approach flow velocity is reduced to zero at the upstream side of the pier, which results in a pressure increase at the pier face. The associated pressures are highest near the surface, where the deceleration is greatest, and decrease downwards. Since the velocity is decreasing from the surface to the bed, the pressure on the face of the pier also decreases accordingly forming a downward pressure gradient. The pressure gradient hence forces the flow down the face of the pier, resembling that of a vertical jet. The resulting downflow impinges on the streambed and creates a hole in the vicinity of the pier base. The downflow impinging on the bed is the main scouring agent. Figure 2 shows the flow and scour pattern at a circular pier.
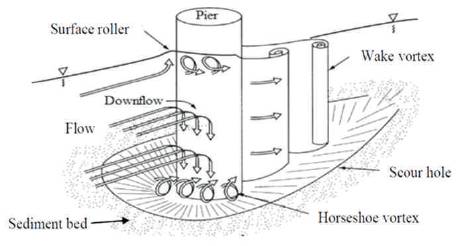
Figure 2. Flow Pattern and Mechanism of Scouring around Bridge Pier
The following factors that affect the local scour depth around piers and abutments are (i) velocity of the approach flow, (ii) depth of flow, (iii) width of the pier, (iv) length of the cylindrical bridge pier if skewed to flow, (v) size and gradation of bed material, (vi) angle of attack to a pier or abutment, (vii) shape of a pier or abutment, (viii) bed configuration, and (ix) ice formation or jams and debris which is not a factor in the present study. These factors have been discussed in the following sections.
The relation between the depths of local scour at a bridge pier Ds and its dependent parameters can be written as

where ρ and v = fluid density and kinematic viscosity, respectively; U = mean approach flow velocity; H = mean approach flow depth; g = acceleration of gravity; d50 and σg= median size and geometric standard deviation of the sediment particle size distribution; ρs= sediment density; D = pier width; t = time; and f denotes “a function of.”and ρ, ρs, U, d50 , D, H, g parameters may be also replaced by the following ones.
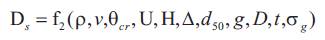
where,Δ =(ρs - ρ) / ρ, the relative submerged density and θCr is Shields' sediment grain entrainment parameter i.e. θCr = U2*c / Δgd50 and U*c is the critical shear velocity at which sediment grain starts moving.
Scour involves three fundamental dimensions i.e. mass, length and time. The eleven independent variables (Eq. 1) are reducible to a set of eight non-dimensional parameters by the Vaschy-Buckingham-theorem. We can also write Eq. (2) in a non-dimensional form as:
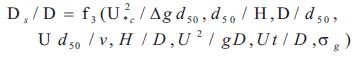
For the flow conditions in the present study, the general statement can be simplified by the following assumptions:
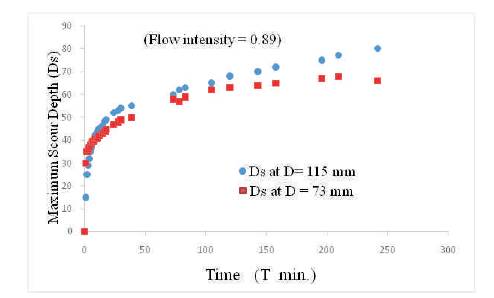
Figure 3. Maximum Scour Depth Vs. Time (D= 115 and 73 mm)
In the present study, D/d50 and clear-water flow condition have been considered. Also the effect of sediment size has been considered in Shields' entrainment parameter θcr= U2*c/ Δgd50 . Thus the non- dimensional parameters d50 / H, D/d50, Ud 50/ υ can be dropped out.
Thus from the above discussion following nondimensional parameters describing the equilibrium scour depth around circular bridge piers at any time, t has been found to be important and can be written as;
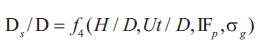
Raudkivi and Ettema (1983) and ShriRam and Pande B.B (1997)derived an equation for estimating the maximum depth of local scour at circular piers based on laboratory experiments for cohesionless bed sediment. They concluded that the equilibrium depth of local scour (Dse) decreased as the geometric standard deviation of sediment (σg) increased (an exception occurs when σg < 1.4. Also observed by Pagliara (2007), in the case of a non-uniform material, i.e., σg < 1.4, the scour is less compared with that of a uniform material with the same median particle size (d50).
Figure 4 shows that the Scour depth is dependant on geometric standard deviation of sediment (σg), as the geometric standard deviation of a sediment mixture increases, the rate of scour decreases the quantitative relationships for the effect of geometric sediment gradation. They concluded that the equilibrium depth of local scour decreased as the geometric standard deviation of sediment increased.
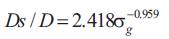
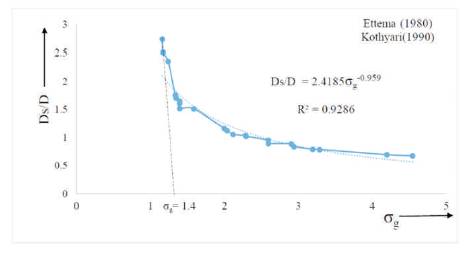
Figure 4. Variation of Non-dimensional Scour Depth (Ds /D) Vs. σg
Analysing the result of fine sand (d50 = 0.16mm) for Figure 5, 100 hours flow duration, the curve of relative scour depth Ds/D versus non-dimensioned time (Ut/D) for different diameter of cylindrical piers are drawn and is shown in Figure 6. It may be observed that the scour depth increases with increasing time, in general.

Figure 5. Variation of Non-dimensional Scour Depth Vs. Pier's Froude Number
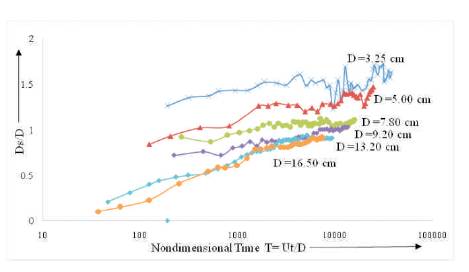
Figure 6. Temporal Variation of Scour Depth Vs. Time
From Figure 7, following equation for scour depth is obtained.
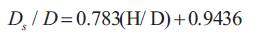
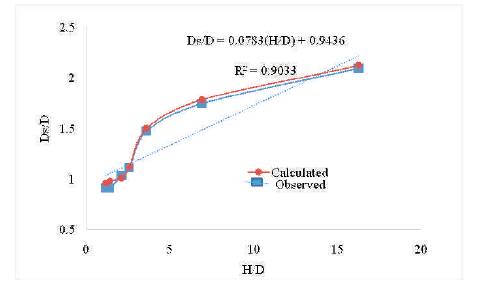
Figure 7. Variation of Non-dimensional Scour Depth Vs. (H/D) in uniform sediment
As an initial attempt to correlate scour depth with time, a curve obtained from regression analysis in functional form-
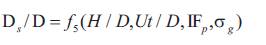
And draping σg for uniform sediments i.e.
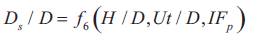
and is plotted in Figure 8. This figure shows that the scour depth is tending to reach equilibrium at long hours of run, also the relationship shows that scour depth varies with time in logarithmic scale in the mid-range.
Figure 8 can be given as the following equation i.e.

where
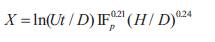
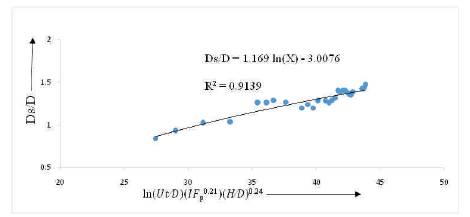
Figure 8. Prediction of Scour Depth as a Function of Time
In the initial and at the final stages, the scour depth follows nonlinear pattern with logarithmic time as variable. This leads to observe the rate of scour as a function of time.
This study is limited to local scouring at cylindrical bridgepiers in uniform and non-uniform sand beds. The following conclusions can be drawn from this study:
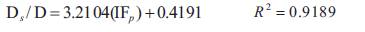

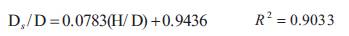

where,
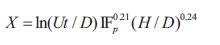
By putting the value of X in above equation, the equation for clear-water local scour depth prediction is achieved.
The following symbols are used in this paper
D = pier diameter (m) ;
Ds= scour depth (m) ;
Dse = equilibrium scour depth (m) ;
d50= median sediment size (m) ;
f = arbitrary function;
g = acceleration due to gravity (m/s2) ;
H = water depth (m) ;
Hs= water depth used to reach the scour depth (m) ;
t = time (s) ;
U = mean approach flow velocity (m/s);
υ= water kinematic viscosity (m2/s) ;
ρ = water density (kg/m3) ;
ρs= sediment density (kg/m3) ;
σg= geometric standard deviation (-)
IFp= pier Froude number of approach flow (-);
Δ= relative submerged density ( ρs-ρ) / ρ;
θcr= Shields' sediment grain entrainment parameter, θcr= U2*c / Δgd50
U*C = critical shear velocity at which sediment grain starts moving.